About the Execution of Marcie for HypertorusGrid-PT-d2k2p1b00
| Execution Summary | |||||
| Max Memory Used (MB) |
Time wait (ms) | CPU Usage (ms) | I/O Wait (ms) | Computed Result | Execution Status |
| 7715.756 | 160006.00 | 160080.00 | 0.00 | FTTTFTTTFTTFTFFF | normal |
Execution Chart
We display below the execution chart for this examination (boot time has been removed).
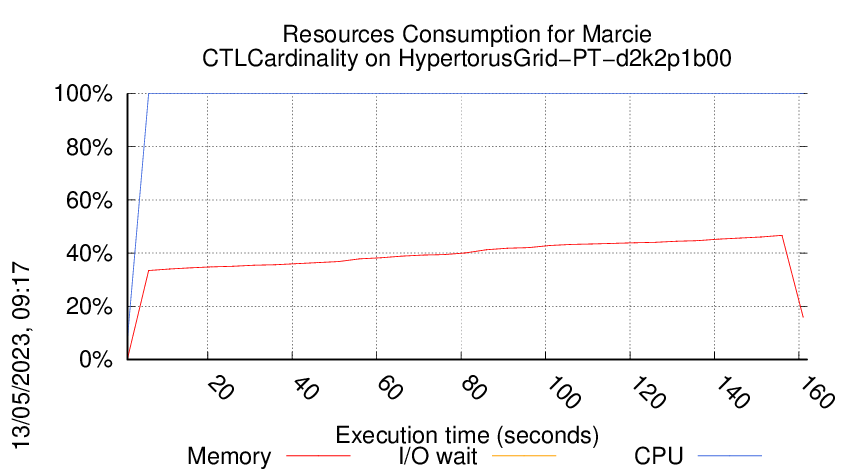
Trace from the execution
Formatting '/data/fkordon/mcc2023-input.r225-tall-167856407000033.qcow2', fmt=qcow2 size=4294967296 backing_file=/data/fkordon/mcc2023-input.qcow2 cluster_size=65536 lazy_refcounts=off refcount_bits=16
Waiting for the VM to be ready (probing ssh)
.................................................................................................
=====================================================================
Generated by BenchKit 2-5348
Executing tool marcie
Input is HypertorusGrid-PT-d2k2p1b00, examination is CTLCardinality
Time confinement is 3600 seconds
Memory confinement is 16384 MBytes
Number of cores is 1
Run identifier is r225-tall-167856407000033
=====================================================================
--------------------
preparation of the directory to be used:
/home/mcc/execution
total 548K
-rw-r--r-- 1 mcc users 10K Feb 26 10:46 CTLCardinality.txt
-rw-r--r-- 1 mcc users 94K Feb 26 10:46 CTLCardinality.xml
-rw-r--r-- 1 mcc users 6.8K Feb 26 10:45 CTLFireability.txt
-rw-r--r-- 1 mcc users 53K Feb 26 10:45 CTLFireability.xml
-rw-r--r-- 1 mcc users 4.2K Jan 29 11:40 GenericPropertiesDefinition.xml
-rw-r--r-- 1 mcc users 6.3K Jan 29 11:40 GenericPropertiesVerdict.xml
-rw-r--r-- 1 mcc users 4.7K Feb 25 16:16 LTLCardinality.txt
-rw-r--r-- 1 mcc users 28K Feb 25 16:16 LTLCardinality.xml
-rw-r--r-- 1 mcc users 3.0K Feb 25 16:17 LTLFireability.txt
-rw-r--r-- 1 mcc users 17K Feb 25 16:17 LTLFireability.xml
-rw-r--r-- 1 mcc users 16K Feb 26 10:47 ReachabilityCardinality.txt
-rw-r--r-- 1 mcc users 144K Feb 26 10:47 ReachabilityCardinality.xml
-rw-r--r-- 1 mcc users 11K Feb 26 10:47 ReachabilityFireability.txt
-rw-r--r-- 1 mcc users 70K Feb 26 10:47 ReachabilityFireability.xml
-rw-r--r-- 1 mcc users 1.9K Feb 25 16:17 UpperBounds.txt
-rw-r--r-- 1 mcc users 3.9K Feb 25 16:17 UpperBounds.xml
-rw-r--r-- 1 mcc users 6 Mar 5 18:22 equiv_col
-rw-r--r-- 1 mcc users 10 Mar 5 18:22 instance
-rw-r--r-- 1 mcc users 6 Mar 5 18:22 iscolored
-rwxr-xr-x 1 mcc users 35K Mar 5 18:22 model.pnml
--------------------
content from stdout:
=== Data for post analysis generated by BenchKit (invocation template)
The expected result is a vector of booleans
BOOL_VECTOR
here is the order used to build the result vector(from text file)
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-00
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-01
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-02
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-03
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-04
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-05
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-06
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-07
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-08
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-09
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-10
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-11
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-12
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-13
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-14
FORMULA_NAME HypertorusGrid-PT-d2k2p1b00-CTLCardinality-15
=== Now, execution of the tool begins
BK_START 1678602362263
bash -c /home/mcc/BenchKit/BenchKit_head.sh 2> STDERR ; echo ; echo -n "BK_STOP " ; date -u +%s%3N
Invoking MCC driver with
BK_TOOL=marcie
BK_EXAMINATION=CTLCardinality
BK_BIN_PATH=/home/mcc/BenchKit/bin/
BK_TIME_CONFINEMENT=3600
BK_INPUT=HypertorusGrid-PT-d2k2p1b00
Not applying reductions.
Model is PT
CTLCardinality PT
timeout --kill-after=10s --signal=SIGINT 1m for testing only
Marcie built on Linux at 2019-11-18.
A model checker for Generalized Stochastic Petri nets
authors: Alex Tovchigrechko (IDD package and CTL model checking)
Martin Schwarick (Symbolic numerical analysis and CSL model checking)
Christian Rohr (Simulative and approximative numerical model checking)
marcie@informatik.tu-cottbus.de
called as: /home/mcc/BenchKit/bin//../marcie/bin/marcie --net-file=model.pnml --mcc-file=CTLCardinality.xml --memory=6 --mcc-mode
parse successfull
net created successfully
Net: HypertorusGrid_PT_d2k2p1b00
(NrP: 52 NrTr: 64 NrArc: 256)
parse formulas
formulas created successfully
place and transition orderings generation:0m 0.000sec
net check time: 0m 0.000sec
init dd package: 0m 2.978sec
RS generation: 0m 0.508sec
-> reachability set: #nodes 1489 (1.5e+03) #states 51,737,129,142 (10)
starting MCC model checker
--------------------------
checking: EX [pil_d2_n1_2_2<=0]
normalized: EX [pil_d2_n1_2_2<=0]
abstracting: (pil_d2_n1_2_2<=0)
states: 18,758,263,731 (10)
.-> the formula is TRUE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-12 TRUE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m 0.054sec
checking: EF [~ [AF [pb_d1_n2_1_2<=pb_d1_n2_2_1]]]
normalized: E [true U EG [~ [pb_d1_n2_1_2<=pb_d1_n2_2_1]]]
abstracting: (pb_d1_n2_1_2<=pb_d1_n2_2_1)
states: 36,456,320,605 (10)
................
EG iterations: 16
-> the formula is TRUE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-10 TRUE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m 2.140sec
checking: AF [EF [AX [AX [E [pi_d1_n1_2_2<=pi_d2_n1_2_1 U 1<=pi_d1_n1_1_2]]]]]
normalized: ~ [EG [~ [E [true U ~ [EX [EX [~ [E [pi_d1_n1_2_2<=pi_d2_n1_2_1 U 1<=pi_d1_n1_1_2]]]]]]]]]
abstracting: (1<=pi_d1_n1_1_2)
states: 18,758,263,731 (10)
abstracting: (pi_d1_n1_2_2<=pi_d2_n1_2_1)
states: 39,505,177,062 (10)
...
EG iterations: 1
-> the formula is TRUE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-03 TRUE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m42.812sec
checking: EG [EX [~ [E [AX [po_d1_n1_1_2<=pb_d2_n2_1_2] U 1<=pi_d2_n1_2_2]]]]
normalized: EG [EX [~ [E [~ [EX [~ [po_d1_n1_1_2<=pb_d2_n2_1_2]]] U 1<=pi_d2_n1_2_2]]]]
abstracting: (1<=pi_d2_n1_2_2)
states: 18,758,263,731 (10)
abstracting: (po_d1_n1_1_2<=pb_d2_n2_1_2)
states: 40,797,750,799 (10)
.........
EG iterations: 7
-> the formula is TRUE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-06 TRUE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m 2.804sec
checking: ~ [[EF [AG [~ [po_d2_n1_2_2<=1]]] & EG [[1<=pb_d1_n2_2_1 & [EX [EF [pol_d1_n1_1_2<=1]] & [~ [pil_d2_n1_1_2<=pbl_2_1] & pb_d1_n1_2_2<=1]]]]]]
normalized: ~ [[EG [[1<=pb_d1_n2_2_1 & [[pb_d1_n1_2_2<=1 & ~ [pil_d2_n1_1_2<=pbl_2_1]] & EX [E [true U pol_d1_n1_1_2<=1]]]]] & E [true U ~ [E [true U po_d2_n1_2_2<=1]]]]]
abstracting: (po_d2_n1_2_2<=1)
states: 51,737,129,142 (10)
abstracting: (pol_d1_n1_1_2<=1)
states: 51,737,129,142 (10)
.abstracting: (pil_d2_n1_1_2<=pbl_2_1)
states: 42,650,384,902 (10)
abstracting: (pb_d1_n1_2_2<=1)
states: 43,641,548,356 (10)
abstracting: (1<=pb_d1_n2_2_1)
states: 22,219,994,884 (10)
.............
EG iterations: 13
-> the formula is TRUE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-01 TRUE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m 0.515sec
checking: AG [AF [[AX [[pb_d1_n1_2_2<=pi_d2_n1_2_2 | AG [po_d2_n1_1_2<=1]]] & A [AF [pol_d2_n1_2_2<=1] U AG [pi_d2_n1_2_1<=pi_d2_n1_1_2]]]]]
normalized: ~ [E [true U EG [~ [[[~ [EG [E [true U ~ [pi_d2_n1_2_1<=pi_d2_n1_1_2]]]] & ~ [E [E [true U ~ [pi_d2_n1_2_1<=pi_d2_n1_1_2]] U [E [true U ~ [pi_d2_n1_2_1<=pi_d2_n1_1_2]] & EG [~ [pol_d2_n1_2_2<=1]]]]]] & ~ [EX [~ [[pb_d1_n1_2_2<=pi_d2_n1_2_2 | ~ [E [true U ~ [po_d2_n1_1_2<=1]]]]]]]]]]]]
abstracting: (po_d2_n1_1_2<=1)
states: 51,737,129,142 (10)
abstracting: (pb_d1_n1_2_2<=pi_d2_n1_2_2)
states: 34,569,521,828 (10)
.abstracting: (pol_d2_n1_2_2<=1)
states: 51,737,129,142 (10)
.
EG iterations: 1
abstracting: (pi_d2_n1_2_1<=pi_d2_n1_1_2)
states: 39,505,177,062 (10)
abstracting: (pi_d2_n1_2_1<=pi_d2_n1_1_2)
states: 39,505,177,062 (10)
abstracting: (pi_d2_n1_2_1<=pi_d2_n1_1_2)
states: 39,505,177,062 (10)
.....
EG iterations: 5
.
EG iterations: 1
-> the formula is FALSE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-14 FALSE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m 0.810sec
checking: EF [EF [[[pi_d1_n1_1_2<=pi_d2_n1_2_1 & AF [AX [1<=pol_d1_n1_2_1]]] & A [~ [1<=pbl_1_2] U ~ [[pil_d2_n1_2_2<=pb_d1_n1_1_1 & pol_d1_n1_1_1<=pil_d2_n1_1_2]]]]]]
normalized: E [true U E [true U [[pi_d1_n1_1_2<=pi_d2_n1_2_1 & ~ [EG [EX [~ [1<=pol_d1_n1_2_1]]]]] & [~ [EG [[pil_d2_n1_2_2<=pb_d1_n1_1_1 & pol_d1_n1_1_1<=pil_d2_n1_1_2]]] & ~ [E [[pil_d2_n1_2_2<=pb_d1_n1_1_1 & pol_d1_n1_1_1<=pil_d2_n1_1_2] U [1<=pbl_1_2 & [pil_d2_n1_2_2<=pb_d1_n1_1_1 & pol_d1_n1_1_1<=pil_d2_n1_1_2]]]]]]]]
abstracting: (pol_d1_n1_1_1<=pil_d2_n1_1_2)
states: 39,505,177,062 (10)
abstracting: (pil_d2_n1_2_2<=pb_d1_n1_1_1)
states: 33,159,373,227 (10)
abstracting: (1<=pbl_1_2)
states: 38,293,230,667 (10)
abstracting: (pol_d1_n1_1_1<=pil_d2_n1_1_2)
states: 39,505,177,062 (10)
abstracting: (pil_d2_n1_2_2<=pb_d1_n1_1_1)
states: 33,159,373,227 (10)
abstracting: (pol_d1_n1_1_1<=pil_d2_n1_1_2)
states: 39,505,177,062 (10)
abstracting: (pil_d2_n1_2_2<=pb_d1_n1_1_1)
states: 33,159,373,227 (10)
..............
EG iterations: 14
abstracting: (1<=pol_d1_n1_2_1)
states: 32,978,865,411 (10)
.......
EG iterations: 6
abstracting: (pi_d1_n1_1_2<=pi_d2_n1_2_1)
states: 39,505,177,062 (10)
-> the formula is TRUE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-07 TRUE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m 7.067sec
checking: AF [[EF [[[E [1<=pi_d1_n1_2_1 U pol_d1_n1_2_1<=pi_d2_n1_1_1] | AG [po_d2_n1_2_1<=pb_d2_n1_2_1]] | AX [~ [pbl_1_2<=0]]]] & ~ [EX [[AF [pi_d2_n1_1_2<=pb_d1_n2_2_2] & EG [pol_d1_n1_1_2<=0]]]]]]
normalized: ~ [EG [~ [[E [true U [[E [1<=pi_d1_n1_2_1 U pol_d1_n1_2_1<=pi_d2_n1_1_1] | ~ [E [true U ~ [po_d2_n1_2_1<=pb_d2_n1_2_1]]]] | ~ [EX [pbl_1_2<=0]]]] & ~ [EX [[EG [pol_d1_n1_1_2<=0] & ~ [EG [~ [pi_d2_n1_1_2<=pb_d1_n2_2_2]]]]]]]]]]
abstracting: (pi_d2_n1_1_2<=pb_d1_n2_2_2)
states: 40,797,750,799 (10)
................
EG iterations: 16
abstracting: (pol_d1_n1_1_2<=0)
states: 18,758,263,731 (10)
.............
EG iterations: 13
.abstracting: (pbl_1_2<=0)
states: 13,443,898,475 (10)
.abstracting: (po_d2_n1_2_1<=pb_d2_n1_2_1)
states: 40,797,750,799 (10)
abstracting: (pol_d1_n1_2_1<=pi_d2_n1_1_1)
states: 30,990,215,811 (10)
abstracting: (1<=pi_d1_n1_2_1)
states: 18,758,263,731 (10)
.........
EG iterations: 9
-> the formula is FALSE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-00 FALSE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m 3.894sec
checking: EF [AG [[EX [E [pil_d1_n1_2_1<=pil_d2_n1_1_1 U pi_d1_n1_1_1<=1]] | A [~ [E [pol_d1_n1_2_1<=pb_d2_n1_2_2 U po_d2_n1_1_2<=1]] U [1<=pb_d2_n1_1_1 & A [pb_d2_n2_1_2<=pil_d1_n1_1_2 U pil_d1_n1_2_2<=0]]]]]]
normalized: E [true U ~ [E [true U ~ [[EX [E [pil_d1_n1_2_1<=pil_d2_n1_1_1 U pi_d1_n1_1_1<=1]] | [~ [EG [~ [[1<=pb_d2_n1_1_1 & [~ [EG [~ [pil_d1_n1_2_2<=0]]] & ~ [E [~ [pil_d1_n1_2_2<=0] U [~ [pb_d2_n2_1_2<=pil_d1_n1_1_2] & ~ [pil_d1_n1_2_2<=0]]]]]]]]] & ~ [E [~ [[1<=pb_d2_n1_1_1 & [~ [EG [~ [pil_d1_n1_2_2<=0]]] & ~ [E [~ [pil_d1_n1_2_2<=0] U [~ [pb_d2_n2_1_2<=pil_d1_n1_1_2] & ~ [pil_d1_n1_2_2<=0]]]]]]] U [E [pol_d1_n1_2_1<=pb_d2_n1_2_2 U po_d2_n1_1_2<=1] & ~ [[1<=pb_d2_n1_1_1 & [~ [EG [~ [pil_d1_n1_2_2<=0]]] & ~ [E [~ [pil_d1_n1_2_2<=0] U [~ [pb_d2_n2_1_2<=pil_d1_n1_1_2] & ~ [pil_d1_n1_2_2<=0]]]]]]]]]]]]]]]]
abstracting: (pil_d1_n1_2_2<=0)
states: 18,758,263,731 (10)
abstracting: (pb_d2_n2_1_2<=pil_d1_n1_1_2)
states: 38,589,160,786 (10)
abstracting: (pil_d1_n1_2_2<=0)
states: 18,758,263,731 (10)
abstracting: (pil_d1_n1_2_2<=0)
states: 18,758,263,731 (10)
.............
EG iterations: 13
abstracting: (1<=pb_d2_n1_1_1)
states: 22,219,994,884 (10)
abstracting: (po_d2_n1_1_2<=1)
states: 51,737,129,142 (10)
abstracting: (pol_d1_n1_2_1<=pb_d2_n1_2_2)
states: 33,159,373,227 (10)
abstracting: (pil_d1_n1_2_2<=0)
states: 18,758,263,731 (10)
abstracting: (pb_d2_n2_1_2<=pil_d1_n1_1_2)
states: 38,589,160,786 (10)
abstracting: (pil_d1_n1_2_2<=0)
states: 18,758,263,731 (10)
abstracting: (pil_d1_n1_2_2<=0)
states: 18,758,263,731 (10)
.............
EG iterations: 13
abstracting: (1<=pb_d2_n1_1_1)
states: 22,219,994,884 (10)
abstracting: (pil_d1_n1_2_2<=0)
states: 18,758,263,731 (10)
abstracting: (pb_d2_n2_1_2<=pil_d1_n1_1_2)
states: 38,589,160,786 (10)
abstracting: (pil_d1_n1_2_2<=0)
states: 18,758,263,731 (10)
abstracting: (pil_d1_n1_2_2<=0)
states: 18,758,263,731 (10)
.............
EG iterations: 13
abstracting: (1<=pb_d2_n1_1_1)
states: 22,219,994,884 (10)
......
EG iterations: 6
abstracting: (pi_d1_n1_1_1<=1)
states: 51,737,129,142 (10)
abstracting: (pil_d1_n1_2_1<=pil_d2_n1_1_1)
states: 39,505,177,062 (10)
.-> the formula is TRUE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-09 TRUE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m14.233sec
checking: AG [[E [E [E [1<=pb_d1_n2_1_2 U po_d2_n1_1_2<=0] U ~ [[pi_d2_n1_1_2<=pil_d1_n1_2_1 | pb_d1_n2_1_2<=0]]] U AF [~ [1<=pb_d1_n1_2_1]]] & AF [~ [[~ [[pb_d1_n2_1_1<=pi_d2_n1_1_1 & 1<=pil_d1_n1_2_2]] & A [pil_d2_n1_1_2<=1 U pil_d2_n1_1_1<=po_d1_n1_2_1]]]]]]
normalized: ~ [E [true U ~ [[~ [EG [[[~ [EG [~ [pil_d2_n1_1_1<=po_d1_n1_2_1]]] & ~ [E [~ [pil_d2_n1_1_1<=po_d1_n1_2_1] U [~ [pil_d2_n1_1_2<=1] & ~ [pil_d2_n1_1_1<=po_d1_n1_2_1]]]]] & ~ [[pb_d1_n2_1_1<=pi_d2_n1_1_1 & 1<=pil_d1_n1_2_2]]]]] & E [E [E [1<=pb_d1_n2_1_2 U po_d2_n1_1_2<=0] U ~ [[pi_d2_n1_1_2<=pil_d1_n1_2_1 | pb_d1_n2_1_2<=0]]] U ~ [EG [1<=pb_d1_n1_2_1]]]]]]]
abstracting: (1<=pb_d1_n1_2_1)
states: 22,219,994,884 (10)
......
EG iterations: 6
abstracting: (pb_d1_n2_1_2<=0)
states: 29,517,134,258 (10)
abstracting: (pi_d2_n1_1_2<=pil_d1_n1_2_1)
states: 45,210,817,491 (10)
abstracting: (po_d2_n1_1_2<=0)
states: 32,978,865,411 (10)
abstracting: (1<=pb_d1_n2_1_2)
states: 22,219,994,884 (10)
abstracting: (1<=pil_d1_n1_2_2)
states: 32,978,865,411 (10)
abstracting: (pb_d1_n2_1_1<=pi_d2_n1_1_1)
states: 34,569,521,828 (10)
abstracting: (pil_d2_n1_1_1<=po_d1_n1_2_1)
states: 30,990,215,811 (10)
abstracting: (pil_d2_n1_1_2<=1)
states: 51,737,129,142 (10)
abstracting: (pil_d2_n1_1_1<=po_d1_n1_2_1)
states: 30,990,215,811 (10)
abstracting: (pil_d2_n1_1_1<=po_d1_n1_2_1)
states: 30,990,215,811 (10)
................
EG iterations: 16
.............
EG iterations: 13
-> the formula is FALSE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-13 FALSE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m16.702sec
checking: ~ [[AG [~ [[[AG [1<=pi_d1_n1_2_1] & EG [pb_d1_n1_2_1<=pil_d2_n1_2_1]] | po_d1_n1_1_1<=0]]] | EX [[[[EF [pol_d1_n1_2_2<=pi_d1_n1_2_1] & AG [pbl_1_1<=pol_d1_n1_2_1]] & AX [EF [pil_d1_n1_2_1<=pi_d2_n1_2_1]]] | [A [EX [1<=pb_d1_n1_1_1] U [1<=pb_d2_n1_1_2 & pb_d2_n2_1_1<=0]] | 1<=pi_d2_n1_1_2]]]]]
normalized: ~ [[~ [E [true U [po_d1_n1_1_1<=0 | [EG [pb_d1_n1_2_1<=pil_d2_n1_2_1] & ~ [E [true U ~ [1<=pi_d1_n1_2_1]]]]]]] | EX [[[1<=pi_d2_n1_1_2 | [~ [EG [~ [[1<=pb_d2_n1_1_2 & pb_d2_n2_1_1<=0]]]] & ~ [E [~ [[1<=pb_d2_n1_1_2 & pb_d2_n2_1_1<=0]] U [~ [EX [1<=pb_d1_n1_1_1]] & ~ [[1<=pb_d2_n1_1_2 & pb_d2_n2_1_1<=0]]]]]]] | [[~ [E [true U ~ [pbl_1_1<=pol_d1_n1_2_1]]] & E [true U pol_d1_n1_2_2<=pi_d1_n1_2_1]] & ~ [EX [~ [E [true U pil_d1_n1_2_1<=pi_d2_n1_2_1]]]]]]]]]
abstracting: (pil_d1_n1_2_1<=pi_d2_n1_2_1)
states: 30,990,215,811 (10)
.abstracting: (pol_d1_n1_2_2<=pi_d1_n1_2_1)
states: 30,990,215,811 (10)
abstracting: (pbl_1_1<=pol_d1_n1_2_1)
states: 23,106,129,515 (10)
abstracting: (pb_d2_n2_1_1<=0)
states: 29,517,134,258 (10)
abstracting: (1<=pb_d2_n1_1_2)
states: 22,219,994,884 (10)
abstracting: (1<=pb_d1_n1_1_1)
states: 22,219,994,884 (10)
.abstracting: (pb_d2_n2_1_1<=0)
states: 29,517,134,258 (10)
abstracting: (1<=pb_d2_n1_1_2)
states: 22,219,994,884 (10)
abstracting: (pb_d2_n2_1_1<=0)
states: 29,517,134,258 (10)
abstracting: (1<=pb_d2_n1_1_2)
states: 22,219,994,884 (10)
......
EG iterations: 6
abstracting: (1<=pi_d2_n1_1_2)
states: 18,758,263,731 (10)
.abstracting: (1<=pi_d1_n1_2_1)
states: 18,758,263,731 (10)
abstracting: (pb_d1_n1_2_1<=pil_d2_n1_2_1)
states: 38,589,160,786 (10)
..........
EG iterations: 10
abstracting: (po_d1_n1_1_1<=0)
states: 32,978,865,411 (10)
-> the formula is FALSE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-15 FALSE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m 6.228sec
checking: ~ [AG [EX [[[[[pb_d2_n1_2_2<=pb_d2_n1_1_1 & pol_d1_n1_1_2<=0] | [1<=pb_d1_n1_2_1 | pil_d1_n1_2_1<=pol_d1_n1_2_2]] & E [pil_d1_n1_2_1<=pb_d2_n1_1_2 U pi_d1_n1_1_1<=1]] | [[[1<=pol_d2_n1_2_2 & pb_d1_n2_2_2<=pil_d2_n1_2_2] & AG [po_d1_n1_1_1<=1]] & [[pil_d1_n1_1_1<=0 & pil_d2_n1_2_1<=0] & AG [pi_d2_n1_2_1<=0]]]]]]]
normalized: E [true U ~ [EX [[[[~ [E [true U ~ [pi_d2_n1_2_1<=0]]] & [pil_d1_n1_1_1<=0 & pil_d2_n1_2_1<=0]] & [~ [E [true U ~ [po_d1_n1_1_1<=1]]] & [1<=pol_d2_n1_2_2 & pb_d1_n2_2_2<=pil_d2_n1_2_2]]] | [E [pil_d1_n1_2_1<=pb_d2_n1_1_2 U pi_d1_n1_1_1<=1] & [[1<=pb_d1_n1_2_1 | pil_d1_n1_2_1<=pol_d1_n1_2_2] | [pb_d2_n1_2_2<=pb_d2_n1_1_1 & pol_d1_n1_1_2<=0]]]]]]]
abstracting: (pol_d1_n1_1_2<=0)
states: 18,758,263,731 (10)
abstracting: (pb_d2_n1_2_2<=pb_d2_n1_1_1)
states: 36,456,320,605 (10)
abstracting: (pil_d1_n1_2_1<=pol_d1_n1_2_2)
states: 39,505,177,062 (10)
abstracting: (1<=pb_d1_n1_2_1)
states: 22,219,994,884 (10)
abstracting: (pi_d1_n1_1_1<=1)
states: 51,737,129,142 (10)
abstracting: (pil_d1_n1_2_1<=pb_d2_n1_1_2)
states: 33,159,373,227 (10)
abstracting: (pb_d1_n2_2_2<=pil_d2_n1_2_2)
states: 38,589,160,786 (10)
abstracting: (1<=pol_d2_n1_2_2)
states: 32,978,865,411 (10)
abstracting: (po_d1_n1_1_1<=1)
states: 51,737,129,142 (10)
abstracting: (pil_d2_n1_2_1<=0)
states: 18,758,263,731 (10)
abstracting: (pil_d1_n1_1_1<=0)
states: 18,758,263,731 (10)
abstracting: (pi_d2_n1_2_1<=0)
states: 32,978,865,411 (10)
.-> the formula is TRUE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-02 TRUE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m26.183sec
checking: [EX [[pb_d1_n1_1_2<=po_d2_n1_1_2 & [[[EF [pil_d1_n1_1_1<=pi_d2_n1_2_1] | ~ [[pb_d2_n1_2_1<=1 & po_d1_n1_1_1<=pb_d2_n2_2_1]]] | ~ [EX [pi_d2_n1_2_1<=1]]] & E [~ [[pb_d1_n2_1_2<=pil_d1_n1_2_2 | 1<=pb_d2_n1_2_2]] U EF [1<=pb_d2_n1_1_2]]]]] & EX [EX [[EX [EF [pb_d1_n1_2_1<=pb_d1_n1_2_1]] & [AG [pb_d1_n2_2_1<=0] & [pb_d1_n2_1_2<=pol_d2_n1_1_1 & [po_d2_n1_2_1<=1 & pi_d1_n1_1_2<=0]]]]]]]
normalized: [EX [[pb_d1_n1_1_2<=po_d2_n1_1_2 & [[~ [EX [pi_d2_n1_2_1<=1]] | [~ [[pb_d2_n1_2_1<=1 & po_d1_n1_1_1<=pb_d2_n2_2_1]] | E [true U pil_d1_n1_1_1<=pi_d2_n1_2_1]]] & E [~ [[pb_d1_n2_1_2<=pil_d1_n1_2_2 | 1<=pb_d2_n1_2_2]] U E [true U 1<=pb_d2_n1_1_2]]]]] & EX [EX [[[[pb_d1_n2_1_2<=pol_d2_n1_1_1 & [po_d2_n1_2_1<=1 & pi_d1_n1_1_2<=0]] & ~ [E [true U ~ [pb_d1_n2_2_1<=0]]]] & EX [E [true U pb_d1_n1_2_1<=pb_d1_n1_2_1]]]]]]
abstracting: (pb_d1_n1_2_1<=pb_d1_n1_2_1)
states: 51,737,129,142 (10)
.abstracting: (pb_d1_n2_2_1<=0)
states: 29,517,134,258 (10)
abstracting: (pi_d1_n1_1_2<=0)
states: 32,978,865,411 (10)
abstracting: (po_d2_n1_2_1<=1)
states: 51,737,129,142 (10)
abstracting: (pb_d1_n2_1_2<=pol_d2_n1_1_1)
states: 38,589,160,786 (10)
..abstracting: (1<=pb_d2_n1_1_2)
states: 22,219,994,884 (10)
abstracting: (1<=pb_d2_n1_2_2)
states: 22,219,994,884 (10)
abstracting: (pb_d1_n2_1_2<=pil_d1_n1_2_2)
states: 38,589,160,786 (10)
abstracting: (pil_d1_n1_1_1<=pi_d2_n1_2_1)
states: 30,990,215,811 (10)
abstracting: (po_d1_n1_1_1<=pb_d2_n2_2_1)
states: 40,797,750,799 (10)
abstracting: (pb_d2_n1_2_1<=1)
states: 43,641,548,356 (10)
abstracting: (pi_d2_n1_2_1<=1)
states: 51,737,129,142 (10)
.abstracting: (pb_d1_n1_1_2<=po_d2_n1_1_2)
states: 34,569,521,828 (10)
.-> the formula is FALSE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-08 FALSE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m 3.064sec
checking: EX [[AG [~ [AF [pb_d1_n1_2_2<=pol_d2_n1_2_1]]] | [[[A [1<=pb_d1_n2_1_1 U EF [pol_d1_n1_2_2<=pil_d2_n1_1_2]] & EX [A [1<=pil_d1_n1_1_2 U 1<=pol_d2_n1_1_2]]] & [~ [[EF [pbl_1_2<=po_d1_n1_1_2] | 1<=pol_d2_n1_1_1]] | pb_d1_n2_2_1<=1]] & [1<=pil_d1_n1_2_1 & [[1<=po_d2_n1_2_1 & EF [1<=pil_d1_n1_2_1]] & ~ [[[1<=pil_d1_n1_1_1 | 1<=pb_d1_n1_1_2] & [pi_d1_n1_2_1<=1 & pol_d2_n1_1_2<=pb_d1_n1_1_2]]]]]]]]
normalized: EX [[[[1<=pil_d1_n1_2_1 & [~ [[[pi_d1_n1_2_1<=1 & pol_d2_n1_1_2<=pb_d1_n1_1_2] & [1<=pil_d1_n1_1_1 | 1<=pb_d1_n1_1_2]]] & [1<=po_d2_n1_2_1 & E [true U 1<=pil_d1_n1_2_1]]]] & [[pb_d1_n2_2_1<=1 | ~ [[1<=pol_d2_n1_1_1 | E [true U pbl_1_2<=po_d1_n1_1_2]]]] & [EX [[~ [EG [~ [1<=pol_d2_n1_1_2]]] & ~ [E [~ [1<=pol_d2_n1_1_2] U [~ [1<=pil_d1_n1_1_2] & ~ [1<=pol_d2_n1_1_2]]]]]] & [~ [EG [~ [E [true U pol_d1_n1_2_2<=pil_d2_n1_1_2]]]] & ~ [E [~ [E [true U pol_d1_n1_2_2<=pil_d2_n1_1_2]] U [~ [1<=pb_d1_n2_1_1] & ~ [E [true U pol_d1_n1_2_2<=pil_d2_n1_1_2]]]]]]]]] | ~ [E [true U ~ [EG [~ [pb_d1_n1_2_2<=pol_d2_n1_2_1]]]]]]]
abstracting: (pb_d1_n1_2_2<=pol_d2_n1_2_1)
states: 38,589,160,786 (10)
.......
EG iterations: 7
abstracting: (pol_d1_n1_2_2<=pil_d2_n1_1_2)
states: 39,505,177,062 (10)
abstracting: (1<=pb_d1_n2_1_1)
states: 22,219,994,884 (10)
abstracting: (pol_d1_n1_2_2<=pil_d2_n1_1_2)
states: 39,505,177,062 (10)
abstracting: (pol_d1_n1_2_2<=pil_d2_n1_1_2)
states: 39,505,177,062 (10)
.
EG iterations: 1
abstracting: (1<=pol_d2_n1_1_2)
states: 32,978,865,411 (10)
abstracting: (1<=pil_d1_n1_1_2)
states: 32,978,865,411 (10)
abstracting: (1<=pol_d2_n1_1_2)
states: 32,978,865,411 (10)
abstracting: (1<=pol_d2_n1_1_2)
states: 32,978,865,411 (10)
.............
EG iterations: 13
.abstracting: (pbl_1_2<=po_d1_n1_1_2)
states: 18,636,323,755 (10)
abstracting: (1<=pol_d2_n1_1_1)
states: 32,978,865,411 (10)
abstracting: (pb_d1_n2_2_1<=1)
states: 43,641,548,356 (10)
abstracting: (1<=pil_d1_n1_2_1)
states: 32,978,865,411 (10)
abstracting: (1<=po_d2_n1_2_1)
states: 18,758,263,731 (10)
abstracting: (1<=pb_d1_n1_1_2)
states: 22,219,994,884 (10)
abstracting: (1<=pil_d1_n1_1_1)
states: 32,978,865,411 (10)
abstracting: (pol_d2_n1_1_2<=pb_d1_n1_1_2)
states: 33,159,373,227 (10)
abstracting: (pi_d1_n1_2_1<=1)
states: 51,737,129,142 (10)
abstracting: (1<=pil_d1_n1_2_1)
states: 32,978,865,411 (10)
.-> the formula is FALSE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-11 FALSE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m 7.824sec
checking: EX [[AG [[[[[pb_d2_n1_2_1<=0 & pb_d2_n1_2_2<=pil_d1_n1_2_2] & AX [1<=pi_d2_n1_1_1]] & [AF [pi_d1_n1_2_1<=pb_d2_n2_2_1] & po_d1_n1_1_2<=0]] | E [[1<=pbl_1_1 | 1<=pb_d2_n2_1_1] U ~ [pbl_1_1<=0]]]] & [[[[E [po_d1_n1_2_2<=pbl_2_2 U 1<=pi_d1_n1_2_1] | [pil_d2_n1_1_1<=pb_d1_n1_2_2 & ~ [1<=pol_d1_n1_1_1]]] | E [[pbl_2_1<=pol_d2_n1_2_1 & pb_d2_n1_1_2<=1] U AF [pil_d1_n1_1_2<=1]]] & [AF [[pb_d1_n1_1_1<=pb_d1_n2_2_2 | 1<=pb_d2_n2_1_1]] | [[[pb_d1_n2_2_1<=1 & pbl_2_2<=1] & A [po_d2_n1_2_1<=pb_d2_n1_2_2 U pb_d1_n1_1_2<=0]] & [EG [1<=pbl_2_2] | AX [1<=pol_d2_n1_2_2]]]]] & ~ [[1<=pil_d2_n1_2_1 & 1<=pol_d1_n1_2_2]]]]]
normalized: EX [[[~ [[1<=pil_d2_n1_2_1 & 1<=pol_d1_n1_2_2]] & [[~ [EG [~ [[pb_d1_n1_1_1<=pb_d1_n2_2_2 | 1<=pb_d2_n2_1_1]]]] | [[[~ [E [~ [pb_d1_n1_1_2<=0] U [~ [po_d2_n1_2_1<=pb_d2_n1_2_2] & ~ [pb_d1_n1_1_2<=0]]]] & ~ [EG [~ [pb_d1_n1_1_2<=0]]]] & [pb_d1_n2_2_1<=1 & pbl_2_2<=1]] & [EG [1<=pbl_2_2] | ~ [EX [~ [1<=pol_d2_n1_2_2]]]]]] & [E [[pbl_2_1<=pol_d2_n1_2_1 & pb_d2_n1_1_2<=1] U ~ [EG [~ [pil_d1_n1_1_2<=1]]]] | [[pil_d2_n1_1_1<=pb_d1_n1_2_2 & ~ [1<=pol_d1_n1_1_1]] | E [po_d1_n1_2_2<=pbl_2_2 U 1<=pi_d1_n1_2_1]]]]] & ~ [E [true U ~ [[E [[1<=pbl_1_1 | 1<=pb_d2_n2_1_1] U ~ [pbl_1_1<=0]] | [[po_d1_n1_1_2<=0 & ~ [EG [~ [pi_d1_n1_2_1<=pb_d2_n2_2_1]]]] & [~ [EX [~ [1<=pi_d2_n1_1_1]]] & [pb_d2_n1_2_1<=0 & pb_d2_n1_2_2<=pil_d1_n1_2_2]]]]]]]]]
abstracting: (pb_d2_n1_2_2<=pil_d1_n1_2_2)
states: 38,589,160,786 (10)
abstracting: (pb_d2_n1_2_1<=0)
states: 29,517,134,258 (10)
abstracting: (1<=pi_d2_n1_1_1)
states: 18,758,263,731 (10)
.abstracting: (pi_d1_n1_2_1<=pb_d2_n2_2_1)
states: 40,797,750,799 (10)
..............
EG iterations: 14
abstracting: (po_d1_n1_1_2<=0)
states: 32,978,865,411 (10)
abstracting: (pbl_1_1<=0)
states: 13,443,898,475 (10)
abstracting: (1<=pb_d2_n2_1_1)
states: 22,219,994,884 (10)
abstracting: (1<=pbl_1_1)
states: 38,293,230,667 (10)
abstracting: (1<=pi_d1_n1_2_1)
states: 18,758,263,731 (10)
abstracting: (po_d1_n1_2_2<=pbl_2_2)
states: 47,379,974,907 (10)
abstracting: (1<=pol_d1_n1_1_1)
states: 32,978,865,411 (10)
abstracting: (pil_d2_n1_1_1<=pb_d1_n1_2_2)
states: 33,159,373,227 (10)
abstracting: (pil_d1_n1_1_2<=1)
states: 51,737,129,142 (10)
.
EG iterations: 1
abstracting: (pb_d2_n1_1_2<=1)
states: 43,641,548,356 (10)
abstracting: (pbl_2_1<=pol_d2_n1_2_1)
states: 23,106,129,515 (10)
abstracting: (1<=pol_d2_n1_2_2)
states: 32,978,865,411 (10)
.abstracting: (1<=pbl_2_2)
states: 38,293,230,667 (10)
......
EG iterations: 6
abstracting: (pbl_2_2<=1)
states: 28,298,554,795 (10)
abstracting: (pb_d1_n2_2_1<=1)
states: 43,641,548,356 (10)
abstracting: (pb_d1_n1_1_2<=0)
states: 29,517,134,258 (10)
......
EG iterations: 6
abstracting: (pb_d1_n1_1_2<=0)
states: 29,517,134,258 (10)
abstracting: (po_d2_n1_2_1<=pb_d2_n1_2_2)
states: 40,797,750,799 (10)
abstracting: (pb_d1_n1_1_2<=0)
states: 29,517,134,258 (10)
abstracting: (1<=pb_d2_n2_1_1)
states: 22,219,994,884 (10)
abstracting: (pb_d1_n1_1_1<=pb_d1_n2_2_2)
states: 36,456,320,605 (10)
................
EG iterations: 16
abstracting: (1<=pol_d1_n1_2_2)
states: 32,978,865,411 (10)
abstracting: (1<=pil_d2_n1_2_1)
states: 32,978,865,411 (10)
.-> the formula is FALSE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-04 FALSE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m10.474sec
checking: [EG [[A [[[[pol_d1_n1_1_2<=pb_d2_n2_2_2 & pi_d2_n1_1_1<=1] & [1<=pb_d2_n1_2_1 | 1<=po_d2_n1_2_1]] | [~ [po_d2_n1_2_1<=0] | [pi_d1_n1_1_1<=pol_d2_n1_1_1 | 1<=pi_d2_n1_2_2]]] U [A [pb_d1_n1_1_2<=po_d2_n1_2_2 U pi_d1_n1_2_2<=1] | ~ [AG [pol_d1_n1_1_2<=pb_d1_n2_1_1]]]] & E [1<=pb_d2_n1_1_1 U ~ [AF [1<=pb_d1_n1_2_2]]]]] & [E [[AX [[A [pi_d1_n1_1_1<=1 U pbl_2_1<=pol_d2_n1_2_2] | [pol_d1_n1_2_1<=1 | po_d2_n1_1_1<=pi_d2_n1_1_1]]] | [~ [A [po_d2_n1_1_2<=1 U 1<=pb_d2_n1_1_2]] | pb_d1_n1_1_1<=pb_d1_n1_1_2]] U A [AF [1<=po_d1_n1_1_1] U ~ [[~ [1<=pb_d2_n1_2_2] & A [pb_d1_n1_2_2<=po_d2_n1_1_1 U 1<=pb_d2_n2_2_2]]]]] | A [A [~ [EG [1<=pol_d1_n1_1_1]] U [pi_d1_n1_1_1<=po_d2_n1_1_2 & pol_d2_n1_1_2<=pil_d1_n1_1_1]] U [po_d2_n1_1_1<=pil_d1_n1_1_2 | EF [[[po_d1_n1_1_2<=1 | 1<=pil_d2_n1_2_2] | [pol_d2_n1_2_1<=pbl_2_2 & 1<=pbl_2_2]]]]]]]
normalized: [[[~ [EG [~ [[po_d2_n1_1_1<=pil_d1_n1_1_2 | E [true U [[pol_d2_n1_2_1<=pbl_2_2 & 1<=pbl_2_2] | [po_d1_n1_1_2<=1 | 1<=pil_d2_n1_2_2]]]]]]] & ~ [E [~ [[po_d2_n1_1_1<=pil_d1_n1_1_2 | E [true U [[pol_d2_n1_2_1<=pbl_2_2 & 1<=pbl_2_2] | [po_d1_n1_1_2<=1 | 1<=pil_d2_n1_2_2]]]]] U [~ [[~ [EG [~ [[pi_d1_n1_1_1<=po_d2_n1_1_2 & pol_d2_n1_1_2<=pil_d1_n1_1_1]]]] & ~ [E [~ [[pi_d1_n1_1_1<=po_d2_n1_1_2 & pol_d2_n1_1_2<=pil_d1_n1_1_1]] U [~ [[pi_d1_n1_1_1<=po_d2_n1_1_2 & pol_d2_n1_1_2<=pil_d1_n1_1_1]] & EG [1<=pol_d1_n1_1_1]]]]]] & ~ [[po_d2_n1_1_1<=pil_d1_n1_1_2 | E [true U [[pol_d2_n1_2_1<=pbl_2_2 & 1<=pbl_2_2] | [po_d1_n1_1_2<=1 | 1<=pil_d2_n1_2_2]]]]]]]]] | E [[[pb_d1_n1_1_1<=pb_d1_n1_1_2 | ~ [[~ [EG [~ [1<=pb_d2_n1_1_2]]] & ~ [E [~ [1<=pb_d2_n1_1_2] U [~ [po_d2_n1_1_2<=1] & ~ [1<=pb_d2_n1_1_2]]]]]]] | ~ [EX [~ [[[pol_d1_n1_2_1<=1 | po_d2_n1_1_1<=pi_d2_n1_1_1] | [~ [EG [~ [pbl_2_1<=pol_d2_n1_2_2]]] & ~ [E [~ [pbl_2_1<=pol_d2_n1_2_2] U [~ [pi_d1_n1_1_1<=1] & ~ [pbl_2_1<=pol_d2_n1_2_2]]]]]]]]]] U [~ [EG [[[~ [EG [~ [1<=pb_d2_n2_2_2]]] & ~ [E [~ [1<=pb_d2_n2_2_2] U [~ [pb_d1_n1_2_2<=po_d2_n1_1_1] & ~ [1<=pb_d2_n2_2_2]]]]] & ~ [1<=pb_d2_n1_2_2]]]] & ~ [E [[[~ [EG [~ [1<=pb_d2_n2_2_2]]] & ~ [E [~ [1<=pb_d2_n2_2_2] U [~ [pb_d1_n1_2_2<=po_d2_n1_1_1] & ~ [1<=pb_d2_n2_2_2]]]]] & ~ [1<=pb_d2_n1_2_2]] U [EG [~ [1<=po_d1_n1_1_1]] & [[~ [EG [~ [1<=pb_d2_n2_2_2]]] & ~ [E [~ [1<=pb_d2_n2_2_2] U [~ [pb_d1_n1_2_2<=po_d2_n1_1_1] & ~ [1<=pb_d2_n2_2_2]]]]] & ~ [1<=pb_d2_n1_2_2]]]]]]]] & EG [[E [1<=pb_d2_n1_1_1 U EG [~ [1<=pb_d1_n1_2_2]]] & [~ [EG [~ [[E [true U ~ [pol_d1_n1_1_2<=pb_d1_n2_1_1]] | [~ [EG [~ [pi_d1_n1_2_2<=1]]] & ~ [E [~ [pi_d1_n1_2_2<=1] U [~ [pb_d1_n1_1_2<=po_d2_n1_2_2] & ~ [pi_d1_n1_2_2<=1]]]]]]]]] & ~ [E [~ [[E [true U ~ [pol_d1_n1_1_2<=pb_d1_n2_1_1]] | [~ [EG [~ [pi_d1_n1_2_2<=1]]] & ~ [E [~ [pi_d1_n1_2_2<=1] U [~ [pb_d1_n1_1_2<=po_d2_n1_2_2] & ~ [pi_d1_n1_2_2<=1]]]]]]] U [~ [[[[pi_d1_n1_1_1<=pol_d2_n1_1_1 | 1<=pi_d2_n1_2_2] | ~ [po_d2_n1_2_1<=0]] | [[1<=pb_d2_n1_2_1 | 1<=po_d2_n1_2_1] & [pol_d1_n1_1_2<=pb_d2_n2_2_2 & pi_d2_n1_1_1<=1]]]] & ~ [[E [true U ~ [pol_d1_n1_1_2<=pb_d1_n2_1_1]] | [~ [EG [~ [pi_d1_n1_2_2<=1]]] & ~ [E [~ [pi_d1_n1_2_2<=1] U [~ [pb_d1_n1_1_2<=po_d2_n1_2_2] & ~ [pi_d1_n1_2_2<=1]]]]]]]]]]]]]]
abstracting: (pi_d1_n1_2_2<=1)
states: 51,737,129,142 (10)
abstracting: (pb_d1_n1_1_2<=po_d2_n1_2_2)
states: 34,569,521,828 (10)
abstracting: (pi_d1_n1_2_2<=1)
states: 51,737,129,142 (10)
abstracting: (pi_d1_n1_2_2<=1)
states: 51,737,129,142 (10)
.
EG iterations: 1
abstracting: (pol_d1_n1_1_2<=pb_d1_n2_1_1)
states: 33,159,373,227 (10)
abstracting: (pi_d2_n1_1_1<=1)
states: 51,737,129,142 (10)
abstracting: (pol_d1_n1_1_2<=pb_d2_n2_2_2)
states: 33,159,373,227 (10)
abstracting: (1<=po_d2_n1_2_1)
states: 18,758,263,731 (10)
abstracting: (1<=pb_d2_n1_2_1)
states: 22,219,994,884 (10)
abstracting: (po_d2_n1_2_1<=0)
states: 32,978,865,411 (10)
abstracting: (1<=pi_d2_n1_2_2)
states: 18,758,263,731 (10)
abstracting: (pi_d1_n1_1_1<=pol_d2_n1_1_1)
states: 45,210,817,491 (10)
abstracting: (pi_d1_n1_2_2<=1)
states: 51,737,129,142 (10)
abstracting: (pb_d1_n1_1_2<=po_d2_n1_2_2)
states: 34,569,521,828 (10)
abstracting: (pi_d1_n1_2_2<=1)
states: 51,737,129,142 (10)
abstracting: (pi_d1_n1_2_2<=1)
states: 51,737,129,142 (10)
.
EG iterations: 1
abstracting: (pol_d1_n1_1_2<=pb_d1_n2_1_1)
states: 33,159,373,227 (10)
abstracting: (pi_d1_n1_2_2<=1)
states: 51,737,129,142 (10)
abstracting: (pb_d1_n1_1_2<=po_d2_n1_2_2)
states: 34,569,521,828 (10)
abstracting: (pi_d1_n1_2_2<=1)
states: 51,737,129,142 (10)
abstracting: (pi_d1_n1_2_2<=1)
states: 51,737,129,142 (10)
.
EG iterations: 1
abstracting: (pol_d1_n1_1_2<=pb_d1_n2_1_1)
states: 33,159,373,227 (10)
.
EG iterations: 1
abstracting: (1<=pb_d1_n1_2_2)
states: 22,219,994,884 (10)
.
EG iterations: 1
abstracting: (1<=pb_d2_n1_1_1)
states: 22,219,994,884 (10)
.
EG iterations: 1
abstracting: (1<=pb_d2_n1_2_2)
states: 22,219,994,884 (10)
abstracting: (1<=pb_d2_n2_2_2)
states: 22,219,994,884 (10)
abstracting: (pb_d1_n1_2_2<=po_d2_n1_1_1)
states: 34,569,521,828 (10)
abstracting: (1<=pb_d2_n2_2_2)
states: 22,219,994,884 (10)
abstracting: (1<=pb_d2_n2_2_2)
states: 22,219,994,884 (10)
.
EG iterations: 1
abstracting: (1<=po_d1_n1_1_1)
states: 18,758,263,731 (10)
.............
EG iterations: 13
abstracting: (1<=pb_d2_n1_2_2)
states: 22,219,994,884 (10)
abstracting: (1<=pb_d2_n2_2_2)
states: 22,219,994,884 (10)
abstracting: (pb_d1_n1_2_2<=po_d2_n1_1_1)
states: 34,569,521,828 (10)
abstracting: (1<=pb_d2_n2_2_2)
states: 22,219,994,884 (10)
abstracting: (1<=pb_d2_n2_2_2)
states: 22,219,994,884 (10)
.
EG iterations: 1
abstracting: (1<=pb_d2_n1_2_2)
states: 22,219,994,884 (10)
abstracting: (1<=pb_d2_n2_2_2)
states: 22,219,994,884 (10)
abstracting: (pb_d1_n1_2_2<=po_d2_n1_1_1)
states: 34,569,521,828 (10)
abstracting: (1<=pb_d2_n2_2_2)
states: 22,219,994,884 (10)
abstracting: (1<=pb_d2_n2_2_2)
states: 22,219,994,884 (10)
.
EG iterations: 1
...............
EG iterations: 15
abstracting: (pbl_2_1<=pol_d2_n1_2_2)
states: 23,106,129,515 (10)
abstracting: (pi_d1_n1_1_1<=1)
states: 51,737,129,142 (10)
abstracting: (pbl_2_1<=pol_d2_n1_2_2)
states: 23,106,129,515 (10)
abstracting: (pbl_2_1<=pol_d2_n1_2_2)
states: 23,106,129,515 (10)
........
EG iterations: 8
abstracting: (po_d2_n1_1_1<=pi_d2_n1_1_1)
states: 39,505,177,062 (10)
abstracting: (pol_d1_n1_2_1<=1)
states: 51,737,129,142 (10)
.abstracting: (1<=pb_d2_n1_1_2)
states: 22,219,994,884 (10)
abstracting: (po_d2_n1_1_2<=1)
states: 51,737,129,142 (10)
abstracting: (1<=pb_d2_n1_1_2)
states: 22,219,994,884 (10)
abstracting: (1<=pb_d2_n1_1_2)
states: 22,219,994,884 (10)
.
EG iterations: 1
abstracting: (pb_d1_n1_1_1<=pb_d1_n1_1_2)
states: 36,456,320,605 (10)
abstracting: (1<=pil_d2_n1_2_2)
states: 32,978,865,411 (10)
abstracting: (po_d1_n1_1_2<=1)
states: 51,737,129,142 (10)
abstracting: (1<=pbl_2_2)
states: 38,293,230,667 (10)
abstracting: (pol_d2_n1_2_1<=pbl_2_2)
states: 42,650,384,902 (10)
abstracting: (po_d2_n1_1_1<=pil_d1_n1_1_2)
states: 45,210,817,491 (10)
abstracting: (1<=pol_d1_n1_1_1)
states: 32,978,865,411 (10)
.............
EG iterations: 13
abstracting: (pol_d2_n1_1_2<=pil_d1_n1_1_1)
states: 39,505,177,062 (10)
abstracting: (pi_d1_n1_1_1<=po_d2_n1_1_2)
states: 39,505,177,062 (10)
abstracting: (pol_d2_n1_1_2<=pil_d1_n1_1_1)
states: 39,505,177,062 (10)
abstracting: (pi_d1_n1_1_1<=po_d2_n1_1_2)
states: 39,505,177,062 (10)
abstracting: (pol_d2_n1_1_2<=pil_d1_n1_1_1)
states: 39,505,177,062 (10)
abstracting: (pi_d1_n1_1_1<=po_d2_n1_1_2)
states: 39,505,177,062 (10)
...............
EG iterations: 15
abstracting: (1<=pil_d2_n1_2_2)
states: 32,978,865,411 (10)
abstracting: (po_d1_n1_1_2<=1)
states: 51,737,129,142 (10)
abstracting: (1<=pbl_2_2)
states: 38,293,230,667 (10)
abstracting: (pol_d2_n1_2_1<=pbl_2_2)
states: 42,650,384,902 (10)
abstracting: (po_d2_n1_1_1<=pil_d1_n1_1_2)
states: 45,210,817,491 (10)
abstracting: (1<=pil_d2_n1_2_2)
states: 32,978,865,411 (10)
abstracting: (po_d1_n1_1_2<=1)
states: 51,737,129,142 (10)
abstracting: (1<=pbl_2_2)
states: 38,293,230,667 (10)
abstracting: (pol_d2_n1_2_1<=pbl_2_2)
states: 42,650,384,902 (10)
abstracting: (po_d2_n1_1_1<=pil_d1_n1_1_2)
states: 45,210,817,491 (10)
.
EG iterations: 1
-> the formula is TRUE
FORMULA HypertorusGrid-PT-d2k2p1b00-CTLCardinality-05 TRUE TECHNIQUES SEQUENTIAL_PROCESSING DECISION_DIAGRAMS UNFOLDING_TO_PT
MC time: 0m 7.830sec
totally nodes used: 27541125 (2.8e+07)
number of garbage collections: 0
fire ops cache: hits/miss/sum: 281535640 291580054 573115694
used/not used/entry size/cache size: 66418221 690643 16 1024MB
basic ops cache: hits/miss/sum: 25694976 26802740 52497716
used/not used/entry size/cache size: 14511840 2265376 12 192MB
unary ops cache: hits/miss/sum: 0 0 0
used/not used/entry size/cache size: 0 16777216 8 128MB
abstract ops cache: hits/miss/sum: 0 0 0
used/not used/entry size/cache size: 0 16777216 12 192MB
state nr cache: hits/miss/sum: 62613 70505 133118
used/not used/entry size/cache size: 70222 8318386 32 256MB
max state cache: hits/miss/sum: 0 0 0
used/not used/entry size/cache size: 0 8388608 32 256MB
uniqueHash elements/entry size/size: 67108864 4 256MB
0 45764608
1 16931051
2 3595349
3 610395
4 108456
5 30362
6 15134
7 9786
8 7555
9 5648
>= 10 30520
Total processing time: 2m39.946sec
BK_STOP 1678602522269
--------------------
content from stderr:
check for maximal unmarked siphon
ok
check for constant places
ok
check if there are places and transitions
ok
check if there are transitions without pre-places
ok
check if at least one transition is enabled in m0
ok
check if there are transitions that can never fire
ok
initing FirstDep: 0m 0.000sec
iterations count:15972 (249), effective:1963 (30)
initing FirstDep: 0m 0.000sec
iterations count:2703 (42), effective:191 (2)
iterations count:3701 (57), effective:225 (3)
iterations count:35270 (551), effective:2585 (40)
iterations count:1519 (23), effective:95 (1)
iterations count:64 (1), effective:0 (0)
iterations count:64 (1), effective:0 (0)
iterations count:3703 (57), effective:229 (3)
iterations count:3703 (57), effective:229 (3)
iterations count:3703 (57), effective:229 (3)
iterations count:64 (1), effective:0 (0)
iterations count:1290 (20), effective:79 (1)
iterations count:12208 (190), effective:895 (13)
iterations count:64 (1), effective:0 (0)
iterations count:5118 (79), effective:302 (4)
iterations count:1700 (26), effective:95 (1)
iterations count:2381 (37), effective:160 (2)
iterations count:5196 (81), effective:337 (5)
iterations count:64 (1), effective:0 (0)
iterations count:5196 (81), effective:337 (5)
iterations count:64 (1), effective:0 (0)
iterations count:5196 (81), effective:337 (5)
iterations count:64 (1), effective:0 (0)
iterations count:38652 (603), effective:2676 (41)
iterations count:24069 (376), effective:1637 (25)
iterations count:757 (11), effective:41 (0)
iterations count:5342 (83), effective:350 (5)
iterations count:2272 (35), effective:219 (3)
iterations count:7803 (121), effective:575 (8)
iterations count:1656 (25), effective:100 (1)
iterations count:1645 (25), effective:93 (1)
iterations count:2580 (40), effective:166 (2)
iterations count:9440 (147), effective:703 (10)
iterations count:985 (15), effective:52 (0)
iterations count:7146 (111), effective:499 (7)
iterations count:64 (1), effective:0 (0)
iterations count:2876 (44), effective:176 (2)
iterations count:38295 (598), effective:2753 (43)
iterations count:64 (1), effective:0 (0)
iterations count:1811 (28), effective:122 (1)
iterations count:1736 (27), effective:106 (1)
iterations count:64 (1), effective:0 (0)
iterations count:2609 (40), effective:158 (2)
iterations count:15390 (240), effective:1177 (18)
iterations count:1419 (22), effective:87 (1)
iterations count:1419 (22), effective:87 (1)
iterations count:128 (2), effective:8 (0)
iterations count:1419 (22), effective:87 (1)
iterations count:2517 (39), effective:159 (2)
iterations count:1751 (27), effective:133 (2)
iterations count:985 (15), effective:52 (0)
iterations count:632 (9), effective:32 (0)
iterations count:10018 (156), effective:726 (11)
iterations count:1393 (21), effective:83 (1)
iterations count:64 (1), effective:0 (0)
iterations count:3469 (54), effective:220 (3)
iterations count:2299 (35), effective:142 (2)
iterations count:2299 (35), effective:142 (2)
iterations count:2299 (35), effective:142 (2)
iterations count:2523 (39), effective:141 (2)
iterations count:3879 (60), effective:242 (3)
iterations count:3879 (60), effective:242 (3)
iterations count:1071 (16), effective:64 (1)
iterations count:3879 (60), effective:242 (3)
iterations count:21876 (341), effective:1521 (23)
iterations count:64 (1), effective:0 (0)
iterations count:789 (12), effective:39 (0)
iterations count:64 (1), effective:0 (0)
iterations count:64 (1), effective:0 (0)
Sequence of Actions to be Executed by the VM
This is useful if one wants to reexecute the tool in the VM from the submitted image disk.
set -x
# this is for BenchKit: configuration of major elements for the test
export BK_INPUT="HypertorusGrid-PT-d2k2p1b00"
export BK_EXAMINATION="CTLCardinality"
export BK_TOOL="marcie"
export BK_RESULT_DIR="/tmp/BK_RESULTS/OUTPUTS"
export BK_TIME_CONFINEMENT="3600"
export BK_MEMORY_CONFINEMENT="16384"
export BK_BIN_PATH="/home/mcc/BenchKit/bin/"
# this is specific to your benchmark or test
export BIN_DIR="$HOME/BenchKit/bin"
# remove the execution directoty if it exists (to avoid increse of .vmdk images)
if [ -d execution ] ; then
rm -rf execution
fi
# this is for BenchKit: explicit launching of the test
echo "====================================================================="
echo " Generated by BenchKit 2-5348"
echo " Executing tool marcie"
echo " Input is HypertorusGrid-PT-d2k2p1b00, examination is CTLCardinality"
echo " Time confinement is $BK_TIME_CONFINEMENT seconds"
echo " Memory confinement is 16384 MBytes"
echo " Number of cores is 1"
echo " Run identifier is r225-tall-167856407000033"
echo "====================================================================="
echo
echo "--------------------"
echo "preparation of the directory to be used:"
tar xzf /home/mcc/BenchKit/INPUTS/HypertorusGrid-PT-d2k2p1b00.tgz
mv HypertorusGrid-PT-d2k2p1b00 execution
cd execution
if [ "CTLCardinality" = "ReachabilityDeadlock" ] || [ "CTLCardinality" = "UpperBounds" ] || [ "CTLCardinality" = "QuasiLiveness" ] || [ "CTLCardinality" = "StableMarking" ] || [ "CTLCardinality" = "Liveness" ] || [ "CTLCardinality" = "OneSafe" ] || [ "CTLCardinality" = "StateSpace" ]; then
rm -f GenericPropertiesVerdict.xml
fi
pwd
ls -lh
echo
echo "--------------------"
echo "content from stdout:"
echo
echo "=== Data for post analysis generated by BenchKit (invocation template)"
echo
if [ "CTLCardinality" = "UpperBounds" ] ; then
echo "The expected result is a vector of positive values"
echo NUM_VECTOR
elif [ "CTLCardinality" != "StateSpace" ] ; then
echo "The expected result is a vector of booleans"
echo BOOL_VECTOR
else
echo "no data necessary for post analysis"
fi
echo
if [ -f "CTLCardinality.txt" ] ; then
echo "here is the order used to build the result vector(from text file)"
for x in $(grep Property CTLCardinality.txt | cut -d ' ' -f 2 | sort -u) ; do
echo "FORMULA_NAME $x"
done
elif [ -f "CTLCardinality.xml" ] ; then # for cunf (txt files deleted;-)
echo echo "here is the order used to build the result vector(from xml file)"
for x in $(grep '
echo "FORMULA_NAME $x"
done
elif [ "CTLCardinality" = "ReachabilityDeadlock" ] || [ "CTLCardinality" = "QuasiLiveness" ] || [ "CTLCardinality" = "StableMarking" ] || [ "CTLCardinality" = "Liveness" ] || [ "CTLCardinality" = "OneSafe" ] ; then
echo "FORMULA_NAME CTLCardinality"
fi
echo
echo "=== Now, execution of the tool begins"
echo
echo -n "BK_START "
date -u +%s%3N
echo
timeout -s 9 $BK_TIME_CONFINEMENT bash -c "/home/mcc/BenchKit/BenchKit_head.sh 2> STDERR ; echo ; echo -n \"BK_STOP \" ; date -u +%s%3N"
if [ $? -eq 137 ] ; then
echo
echo "BK_TIME_CONFINEMENT_REACHED"
fi
echo
echo "--------------------"
echo "content from stderr:"
echo
cat STDERR ;

