About the Execution of ITS-Tools for Sudoku-COL-BN04
| Execution Summary | |||||
| Max Memory Used (MB) |
Time wait (ms) | CPU Usage (ms) | I/O Wait (ms) | Computed Result | Execution Status |
| 718.903 | 28851.00 | 45139.00 | 1341.50 | FTTFTFTFTFTFTTTF | normal |
Execution Chart
We display below the execution chart for this examination (boot time has been removed).
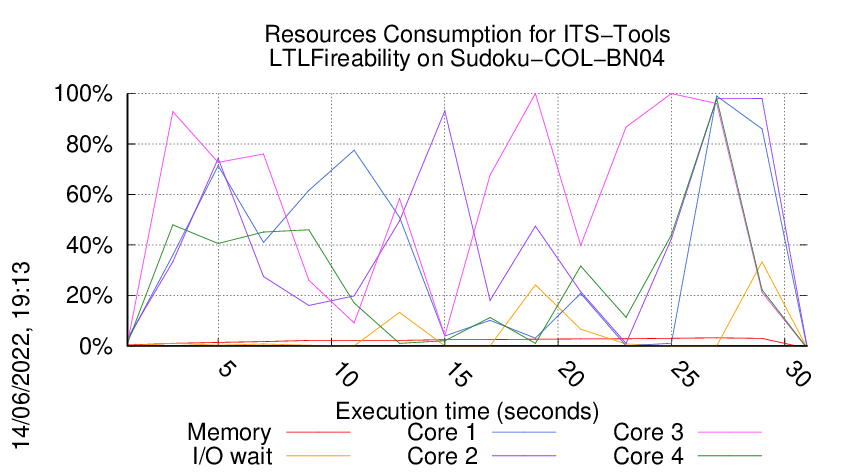
Trace from the execution
Formatting '/data/fkordon/mcc2022-input.r258-tall-165303545400180.qcow2', fmt=qcow2 size=4294967296 backing_file=/data/fkordon/mcc2022-input.qcow2 cluster_size=65536 lazy_refcounts=off refcount_bits=16
Waiting for the VM to be ready (probing ssh)
........................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................
=====================================================================
Generated by BenchKit 2-4028
Executing tool itstools
Input is Sudoku-COL-BN04, examination is LTLFireability
Time confinement is 3600 seconds
Memory confinement is 16384 MBytes
Number of cores is 4
Run identifier is r258-tall-165303545400180
=====================================================================
--------------------
preparation of the directory to be used:
/home/mcc/execution
total 276K
-rw-r--r-- 1 mcc users 6.9K Apr 30 12:25 CTLCardinality.txt
-rw-r--r-- 1 mcc users 69K Apr 30 12:25 CTLCardinality.xml
-rw-r--r-- 1 mcc users 6.6K Apr 30 12:22 CTLFireability.txt
-rw-r--r-- 1 mcc users 62K Apr 30 12:22 CTLFireability.xml
-rw-r--r-- 1 mcc users 4.2K May 10 09:34 GenericPropertiesDefinition.xml
-rw-r--r-- 1 mcc users 6.6K May 10 09:34 GenericPropertiesVerdict.xml
-rw-r--r-- 1 mcc users 3.9K May 9 09:12 LTLCardinality.txt
-rw-r--r-- 1 mcc users 28K May 9 09:12 LTLCardinality.xml
-rw-r--r-- 1 mcc users 2.2K May 9 09:12 LTLFireability.txt
-rw-r--r-- 1 mcc users 16K May 9 09:12 LTLFireability.xml
-rw-r--r-- 1 mcc users 1.6K May 9 09:12 UpperBounds.txt
-rw-r--r-- 1 mcc users 3.7K May 9 09:12 UpperBounds.xml
-rw-r--r-- 1 mcc users 5 May 10 09:34 equiv_pt
-rw-r--r-- 1 mcc users 5 May 10 09:34 instance
-rw-r--r-- 1 mcc users 5 May 10 09:34 iscolored
-rw-r--r-- 1 mcc users 36K May 10 09:34 model.pnml
--------------------
content from stdout:
=== Data for post analysis generated by BenchKit (invocation template)
The expected result is a vector of booleans
BOOL_VECTOR
here is the order used to build the result vector(from text file)
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-00
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-01
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-02
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-03
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-04
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-05
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-06
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-07
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-08
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-09
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-10
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-11
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-12
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-13
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-14
FORMULA_NAME Sudoku-COL-BN04-LTLFireability-15
=== Now, execution of the tool begins
BK_START 1653087936266
Running Version 202205111006
[2022-05-20 23:05:37] [INFO ] Running its-tools with arguments : [-pnfolder, /home/mcc/execution, -examination, LTLFireability, -spotpath, /home/mcc/BenchKit/bin//..//ltlfilt, -z3path, /home/mcc/BenchKit/bin//..//z3/bin/z3, -yices2path, /home/mcc/BenchKit/bin//..//yices/bin/yices, -its, -ltsmin, -greatspnpath, /home/mcc/BenchKit/bin//..//greatspn/, -order, META, -manyOrder, -smt, -timeout, 3600]
[2022-05-20 23:05:37] [INFO ] Parsing pnml file : /home/mcc/execution/model.pnml
[2022-05-20 23:05:37] [INFO ] Detected file is not PT type :http://www.pnml.org/version-2009/grammar/symmetricnet
log4j:WARN No appenders could be found for logger (org.apache.axiom.locator.DefaultOMMetaFactoryLocator).
log4j:WARN Please initialize the log4j system properly.
log4j:WARN See http://logging.apache.org/log4j/1.2/faq.html#noconfig for more info.
[2022-05-20 23:05:37] [WARNING] Using fallBack plugin, rng conformance not checked
[2022-05-20 23:05:38] [INFO ] Load time of PNML (colored model parsed with PNMLFW) : 539 ms
[2022-05-20 23:05:38] [INFO ] Imported 5 HL places and 1 HL transitions for a total of 128 PT places and 256.0 transition bindings in 18 ms.
Parsed 16 properties from file /home/mcc/execution/LTLFireability.xml in 9 ms.
Working with output stream class java.io.PrintStream
[2022-05-20 23:05:38] [INFO ] Built PT skeleton of HLPN with 5 places and 1 transitions 5 arcs in 25 ms.
[2022-05-20 23:05:38] [INFO ] Skeletonized 16 HLPN properties in 1 ms.
Computed a total of 5 stabilizing places and 1 stable transitions
Complete graph has no SCC; deadlocks are unavoidable. place count 5 transition count 1
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge (and all enablings converge to false).
All 16 properties of the HLPN use transition enablings in a way that makes the skeleton too coarse.
Domain [N(4), N(4)] of place Rows breaks symmetries in sort N
[2022-05-20 23:05:38] [INFO ] Unfolded HLPN to a Petri net with 128 places and 64 transitions 320 arcs in 24 ms.
[2022-05-20 23:05:38] [INFO ] Unfolded 16 HLPN properties in 1 ms.
Initial state reduction rules removed 2 formulas.
FORMULA Sudoku-COL-BN04-LTLFireability-06 TRUE TECHNIQUES TOPOLOGICAL INITIAL_STATE
FORMULA Sudoku-COL-BN04-LTLFireability-13 TRUE TECHNIQUES TOPOLOGICAL INITIAL_STATE
FORMULA Sudoku-COL-BN04-LTLFireability-11 FALSE TECHNIQUES TOPOLOGICAL INITIAL_STATE
Support contains 64 out of 128 places. Attempting structural reductions.
Starting structural reductions in LTL mode, iteration 0 : 128/128 places, 64/64 transitions.
Reduce places removed 64 places and 0 transitions.
Iterating post reduction 0 with 64 rules applied. Total rules applied 64 place count 64 transition count 64
Applied a total of 64 rules in 23 ms. Remains 64 /128 variables (removed 64) and now considering 64/64 (removed 0) transitions.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:38] [INFO ] Computed 24 place invariants in 27 ms
[2022-05-20 23:05:38] [INFO ] Implicit Places using invariants in 162 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:38] [INFO ] Computed 24 place invariants in 9 ms
[2022-05-20 23:05:38] [INFO ] Implicit Places using invariants and state equation in 76 ms returned []
Implicit Place search using SMT with State Equation took 282 ms to find 0 implicit places.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:38] [INFO ] Computed 24 place invariants in 2 ms
[2022-05-20 23:05:38] [INFO ] Dead Transitions using invariants and state equation in 71 ms found 0 transitions.
Starting structural reductions in LTL mode, iteration 1 : 64/128 places, 64/64 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/128 places, 64/64 transitions.
Support contains 64 out of 64 places after structural reductions.
[2022-05-20 23:05:39] [INFO ] Flatten gal took : 49 ms
[2022-05-20 23:05:39] [INFO ] Flatten gal took : 59 ms
[2022-05-20 23:05:39] [INFO ] Input system was already deterministic with 64 transitions.
Finished random walk after 12 steps, including 0 resets, run visited all 4 properties in 13 ms. (steps per millisecond=0 )
Computed a total of 64 stabilizing places and 64 stable transitions
Complete graph has no SCC; deadlocks are unavoidable. place count 64 transition count 64
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge (and all enablings converge to false).
Running Spot : cd /home/mcc/execution;'/home/mcc/BenchKit/bin//..//ltl2tgba' '--check=stutter' '--hoaf=tv' '-f' '!(X((X(p0) U !p0)))'
Support contains 64 out of 64 places. Attempting structural reductions.
Starting structural reductions in LTL mode, iteration 0 : 64/64 places, 64/64 transitions.
Applied a total of 0 rules in 1 ms. Remains 64 /64 variables (removed 0) and now considering 64/64 (removed 0) transitions.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:39] [INFO ] Computed 24 place invariants in 2 ms
[2022-05-20 23:05:39] [INFO ] Implicit Places using invariants in 27 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:39] [INFO ] Computed 24 place invariants in 3 ms
[2022-05-20 23:05:39] [INFO ] Implicit Places using invariants and state equation in 60 ms returned []
Implicit Place search using SMT with State Equation took 89 ms to find 0 implicit places.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:39] [INFO ] Computed 24 place invariants in 2 ms
[2022-05-20 23:05:40] [INFO ] Dead Transitions using invariants and state equation in 55 ms found 0 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/64 places, 64/64 transitions.
Stuttering acceptance computed with spot in 192 ms :[p0, p0, true]
Running random walk in product with property : Sudoku-COL-BN04-LTLFireability-00 automaton TGBA Formula[mat=[[{ cond=p0, acceptance={} source=0 dest: 2}], [{ cond=true, acceptance={} source=1 dest: 0}], [{ cond=true, acceptance={0} source=2 dest: 2}]], initial=1, aps=[p0:(OR (AND (GEQ s14 1) (GEQ s30 1) (GEQ s46 1) (GEQ s58 1)) (AND (GEQ s7 1) (GEQ s23 1) (GEQ s38 1) (GEQ s59 1)) (AND (GEQ s15 1) (GEQ s27 1) (GEQ s44 1)...], nbAcceptance=1, properties=[trans-labels, explicit-labels, trans-acc, deterministic, no-univ-branch, unambiguous, semi-deterministic, stutter-sensitive, terminal, very-weak, weak, inherently-weak], stateDesc=[null, null, null][false, false, false]]
Entered a terminal (fully accepting) state of product in 1 steps with 0 reset in 1 ms.
FORMULA Sudoku-COL-BN04-LTLFireability-00 FALSE TECHNIQUES STUTTER_TEST
Treatment of property Sudoku-COL-BN04-LTLFireability-00 finished in 383 ms.
Running Spot : cd /home/mcc/execution;'/home/mcc/BenchKit/bin//..//ltl2tgba' '--check=stutter' '--hoaf=tv' '-f' '!(F(X(F(G(F((F(X(!(p0 U p0)))||!p0)))))))'
Support contains 64 out of 64 places. Attempting structural reductions.
Starting structural reductions in SI_LTL mode, iteration 0 : 64/64 places, 64/64 transitions.
Applied a total of 0 rules in 4 ms. Remains 64 /64 variables (removed 0) and now considering 64/64 (removed 0) transitions.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:40] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:40] [INFO ] Implicit Places using invariants in 30 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:40] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:40] [INFO ] Implicit Places using invariants and state equation in 44 ms returned []
Implicit Place search using SMT with State Equation took 75 ms to find 0 implicit places.
[2022-05-20 23:05:40] [INFO ] Redundant transitions in 1 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:40] [INFO ] Computed 24 place invariants in 3 ms
[2022-05-20 23:05:40] [INFO ] Dead Transitions using invariants and state equation in 81 ms found 0 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/64 places, 64/64 transitions.
Stuttering acceptance computed with spot in 58 ms :[p0, p0]
Running random walk in product with property : Sudoku-COL-BN04-LTLFireability-01 automaton TGBA Formula[mat=[[{ cond=true, acceptance={} source=0 dest: 0}, { cond=p0, acceptance={} source=0 dest: 1}], [{ cond=p0, acceptance={0} source=1 dest: 1}]], initial=0, aps=[p0:(OR (AND (GEQ s14 1) (GEQ s30 1) (GEQ s46 1) (GEQ s58 1)) (AND (GEQ s7 1) (GEQ s23 1) (GEQ s38 1) (GEQ s59 1)) (AND (GEQ s15 1) (GEQ s27 1) (GEQ s44 1)...], nbAcceptance=1, properties=[trans-labels, explicit-labels, trans-acc, no-univ-branch, stutter-invariant, very-weak, weak, inherently-weak], stateDesc=[null, null][true, true]]
Product exploration explored 100000 steps with 7176 reset in 668 ms.
Product exploration explored 100000 steps with 7184 reset in 611 ms.
Computed a total of 64 stabilizing places and 64 stable transitions
Complete graph has no SCC; deadlocks are unavoidable. place count 64 transition count 64
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge (and all enablings converge to false).
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge : F ( (Ga|G!a) & (Gb|G!b)...)
Knowledge obtained : [p0, (X p0), (X (X p0)), (F (G (NOT p0)))]
False Knowledge obtained : []
Property proved to be true thanks to knowledge :(F (G (NOT p0)))
Knowledge based reduction with 4 factoid took 99 ms. Reduced automaton from 2 states, 3 edges and 1 AP to 1 states, 0 edges and 0 AP.
FORMULA Sudoku-COL-BN04-LTLFireability-01 TRUE TECHNIQUES KNOWLEDGE
Treatment of property Sudoku-COL-BN04-LTLFireability-01 finished in 1695 ms.
Running Spot : cd /home/mcc/execution;'/home/mcc/BenchKit/bin//..//ltl2tgba' '--check=stutter' '--hoaf=tv' '-f' '!(X((X((G(p0) U p0)) U X((p0 U p0)))))'
Support contains 64 out of 64 places. Attempting structural reductions.
Starting structural reductions in LTL mode, iteration 0 : 64/64 places, 64/64 transitions.
Applied a total of 0 rules in 0 ms. Remains 64 /64 variables (removed 0) and now considering 64/64 (removed 0) transitions.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:41] [INFO ] Computed 24 place invariants in 3 ms
[2022-05-20 23:05:41] [INFO ] Implicit Places using invariants in 43 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:41] [INFO ] Computed 24 place invariants in 4 ms
[2022-05-20 23:05:42] [INFO ] Implicit Places using invariants and state equation in 48 ms returned []
Implicit Place search using SMT with State Equation took 93 ms to find 0 implicit places.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:42] [INFO ] Computed 24 place invariants in 2 ms
[2022-05-20 23:05:42] [INFO ] Dead Transitions using invariants and state equation in 50 ms found 0 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/64 places, 64/64 transitions.
Stuttering acceptance computed with spot in 113 ms :[(NOT p0), (NOT p0), true, (NOT p0)]
Running random walk in product with property : Sudoku-COL-BN04-LTLFireability-02 automaton TGBA Formula[mat=[[{ cond=(NOT p0), acceptance={} source=0 dest: 2}], [{ cond=true, acceptance={} source=1 dest: 0}], [{ cond=true, acceptance={0} source=2 dest: 2}], [{ cond=true, acceptance={} source=3 dest: 1}]], initial=3, aps=[p0:(OR (AND (GEQ s14 1) (GEQ s30 1) (GEQ s46 1) (GEQ s58 1)) (AND (GEQ s7 1) (GEQ s23 1) (GEQ s38 1) (GEQ s59 1)) (AND (GEQ s15 1) (GEQ s27 1) (GEQ s44 1)...], nbAcceptance=1, properties=[trans-labels, explicit-labels, trans-acc, deterministic, no-univ-branch, unambiguous, semi-deterministic, stutter-sensitive, terminal, very-weak, weak, inherently-weak], stateDesc=[null, null, null, null][false, false, false, false]]
Product exploration explored 100000 steps with 33333 reset in 751 ms.
Product exploration explored 100000 steps with 33333 reset in 725 ms.
Computed a total of 64 stabilizing places and 64 stable transitions
Complete graph has no SCC; deadlocks are unavoidable. place count 64 transition count 64
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge (and all enablings converge to false).
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge : F ( (Ga|G!a) & (Gb|G!b)...)
Knowledge obtained : [p0, (X (X p0)), (F (G (NOT p0)))]
False Knowledge obtained : []
Property proved to be true thanks to knowledge :(X (X p0))
Knowledge based reduction with 3 factoid took 102 ms. Reduced automaton from 4 states, 4 edges and 1 AP to 1 states, 0 edges and 0 AP.
FORMULA Sudoku-COL-BN04-LTLFireability-02 TRUE TECHNIQUES KNOWLEDGE
Treatment of property Sudoku-COL-BN04-LTLFireability-02 finished in 1909 ms.
Running Spot : cd /home/mcc/execution;'/home/mcc/BenchKit/bin//..//ltl2tgba' '--check=stutter' '--hoaf=tv' '-f' '!((F(p0)&&X(F(X(G(F(X(p0))))))&&X(p0)))'
Support contains 64 out of 64 places. Attempting structural reductions.
Starting structural reductions in LTL mode, iteration 0 : 64/64 places, 64/64 transitions.
Applied a total of 0 rules in 1 ms. Remains 64 /64 variables (removed 0) and now considering 64/64 (removed 0) transitions.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:43] [INFO ] Computed 24 place invariants in 5 ms
[2022-05-20 23:05:43] [INFO ] Implicit Places using invariants in 34 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:43] [INFO ] Computed 24 place invariants in 8 ms
[2022-05-20 23:05:43] [INFO ] Implicit Places using invariants and state equation in 47 ms returned []
Implicit Place search using SMT with State Equation took 82 ms to find 0 implicit places.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:43] [INFO ] Computed 24 place invariants in 0 ms
[2022-05-20 23:05:43] [INFO ] Dead Transitions using invariants and state equation in 42 ms found 0 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/64 places, 64/64 transitions.
Stuttering acceptance computed with spot in 131 ms :[(NOT p0), (NOT p0), true, (NOT p0), (NOT p0)]
Running random walk in product with property : Sudoku-COL-BN04-LTLFireability-03 automaton TGBA Formula[mat=[[{ cond=true, acceptance={} source=0 dest: 1}], [{ cond=(NOT p0), acceptance={} source=1 dest: 2}, { cond=p0, acceptance={} source=1 dest: 3}], [{ cond=true, acceptance={0} source=2 dest: 2}], [{ cond=true, acceptance={} source=3 dest: 3}, { cond=(NOT p0), acceptance={} source=3 dest: 4}], [{ cond=(NOT p0), acceptance={0} source=4 dest: 4}]], initial=0, aps=[p0:(OR (AND (GEQ s14 1) (GEQ s30 1) (GEQ s46 1) (GEQ s58 1)) (AND (GEQ s7 1) (GEQ s23 1) (GEQ s38 1) (GEQ s59 1)) (AND (GEQ s15 1) (GEQ s27 1) (GEQ s44 1)...], nbAcceptance=1, properties=[trans-labels, explicit-labels, trans-acc, no-univ-branch, stutter-sensitive, very-weak, weak, inherently-weak], stateDesc=[null, null, null, null, null][false, false, false, false, false]]
Stuttering criterion allowed to conclude after 12 steps with 0 reset in 1 ms.
FORMULA Sudoku-COL-BN04-LTLFireability-03 FALSE TECHNIQUES STUTTER_TEST
Treatment of property Sudoku-COL-BN04-LTLFireability-03 finished in 275 ms.
Running Spot : cd /home/mcc/execution;'/home/mcc/BenchKit/bin//..//ltl2tgba' '--check=stutter' '--hoaf=tv' '-f' '!(F(G((X(F(p1))&&p0))))'
Support contains 64 out of 64 places. Attempting structural reductions.
Starting structural reductions in SI_LTL mode, iteration 0 : 64/64 places, 64/64 transitions.
Applied a total of 0 rules in 2 ms. Remains 64 /64 variables (removed 0) and now considering 64/64 (removed 0) transitions.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:44] [INFO ] Computed 24 place invariants in 2 ms
[2022-05-20 23:05:44] [INFO ] Implicit Places using invariants in 30 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:44] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:44] [INFO ] Implicit Places using invariants and state equation in 46 ms returned []
Implicit Place search using SMT with State Equation took 78 ms to find 0 implicit places.
[2022-05-20 23:05:44] [INFO ] Redundant transitions in 0 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:44] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:44] [INFO ] Dead Transitions using invariants and state equation in 50 ms found 0 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/64 places, 64/64 transitions.
Stuttering acceptance computed with spot in 115 ms :[(OR (NOT p0) (NOT p1)), (NOT p1)]
Running random walk in product with property : Sudoku-COL-BN04-LTLFireability-04 automaton TGBA Formula[mat=[[{ cond=p0, acceptance={} source=0 dest: 0}, { cond=(NOT p0), acceptance={0} source=0 dest: 0}, { cond=(AND p0 (NOT p1)), acceptance={} source=0 dest: 1}], [{ cond=(NOT p1), acceptance={0} source=1 dest: 1}]], initial=0, aps=[p0:(AND (OR (LT s14 1) (LT s30 1) (LT s46 1) (LT s58 1)) (OR (LT s7 1) (LT s23 1) (LT s38 1) (LT s59 1)) (OR (LT s15 1) (LT s27 1) (LT s44 1) (LT s51 1)) ...], nbAcceptance=1, properties=[trans-labels, explicit-labels, trans-acc, no-univ-branch, stutter-invariant], stateDesc=[null, null][true, true]]
Product exploration explored 100000 steps with 7179 reset in 800 ms.
Product exploration explored 100000 steps with 7175 reset in 775 ms.
Computed a total of 64 stabilizing places and 64 stable transitions
Complete graph has no SCC; deadlocks are unavoidable. place count 64 transition count 64
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge (and all enablings converge to false).
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge : F ( (Ga|G!a) & (Gb|G!b)...)
Knowledge obtained : [(AND (NOT p0) (NOT p1)), (X (NOT (AND p0 (NOT p1)))), (X (NOT p1)), (X (NOT p0)), (X (X (NOT (AND p0 (NOT p1))))), (X (X (NOT p1))), (X (X (NOT p0))), (F (G p0)), (F (G p1))]
False Knowledge obtained : []
Property proved to be true thanks to knowledge :(F (G p0))
Knowledge based reduction with 9 factoid took 322 ms. Reduced automaton from 2 states, 4 edges and 2 AP to 1 states, 0 edges and 0 AP.
FORMULA Sudoku-COL-BN04-LTLFireability-04 TRUE TECHNIQUES KNOWLEDGE
Treatment of property Sudoku-COL-BN04-LTLFireability-04 finished in 2222 ms.
Running Spot : cd /home/mcc/execution;'/home/mcc/BenchKit/bin//..//ltl2tgba' '--check=stutter' '--hoaf=tv' '-f' '!((G(F(X(p0)))||G(p0)))'
Support contains 64 out of 64 places. Attempting structural reductions.
Starting structural reductions in SI_LTL mode, iteration 0 : 64/64 places, 64/64 transitions.
Applied a total of 0 rules in 2 ms. Remains 64 /64 variables (removed 0) and now considering 64/64 (removed 0) transitions.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:46] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:46] [INFO ] Implicit Places using invariants in 32 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:46] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:46] [INFO ] Implicit Places using invariants and state equation in 47 ms returned []
Implicit Place search using SMT with State Equation took 81 ms to find 0 implicit places.
[2022-05-20 23:05:46] [INFO ] Redundant transitions in 0 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:46] [INFO ] Computed 24 place invariants in 0 ms
[2022-05-20 23:05:46] [INFO ] Dead Transitions using invariants and state equation in 47 ms found 0 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/64 places, 64/64 transitions.
Stuttering acceptance computed with spot in 60 ms :[(NOT p0), (NOT p0)]
Running random walk in product with property : Sudoku-COL-BN04-LTLFireability-05 automaton TGBA Formula[mat=[[{ cond=true, acceptance={} source=0 dest: 0}, { cond=(NOT p0), acceptance={} source=0 dest: 1}], [{ cond=(NOT p0), acceptance={0} source=1 dest: 1}]], initial=0, aps=[p0:(OR (AND (GEQ s14 1) (GEQ s30 1) (GEQ s46 1) (GEQ s58 1)) (AND (GEQ s7 1) (GEQ s23 1) (GEQ s38 1) (GEQ s59 1)) (AND (GEQ s15 1) (GEQ s27 1) (GEQ s44 1)...], nbAcceptance=1, properties=[trans-labels, explicit-labels, trans-acc, no-univ-branch, stutter-invariant, very-weak, weak, inherently-weak], stateDesc=[null, null][true, true]]
Stuttering criterion allowed to conclude after 12 steps with 0 reset in 1 ms.
FORMULA Sudoku-COL-BN04-LTLFireability-05 FALSE TECHNIQUES STUTTER_TEST
Treatment of property Sudoku-COL-BN04-LTLFireability-05 finished in 209 ms.
Running Spot : cd /home/mcc/execution;'/home/mcc/BenchKit/bin//..//ltl2tgba' '--check=stutter' '--hoaf=tv' '-f' '!((X(G(p0))&&F(X(X(p0)))))'
Support contains 64 out of 64 places. Attempting structural reductions.
Starting structural reductions in LTL mode, iteration 0 : 64/64 places, 64/64 transitions.
Applied a total of 0 rules in 1 ms. Remains 64 /64 variables (removed 0) and now considering 64/64 (removed 0) transitions.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:46] [INFO ] Computed 24 place invariants in 0 ms
[2022-05-20 23:05:46] [INFO ] Implicit Places using invariants in 29 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:46] [INFO ] Computed 24 place invariants in 5 ms
[2022-05-20 23:05:46] [INFO ] Implicit Places using invariants and state equation in 50 ms returned []
Implicit Place search using SMT with State Equation took 81 ms to find 0 implicit places.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:46] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:46] [INFO ] Dead Transitions using invariants and state equation in 46 ms found 0 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/64 places, 64/64 transitions.
Stuttering acceptance computed with spot in 87 ms :[true, (NOT p0), (NOT p0)]
Running random walk in product with property : Sudoku-COL-BN04-LTLFireability-07 automaton TGBA Formula[mat=[[{ cond=true, acceptance={0} source=0 dest: 0}], [{ cond=true, acceptance={} source=1 dest: 2}], [{ cond=(NOT p0), acceptance={} source=2 dest: 0}, { cond=p0, acceptance={} source=2 dest: 2}]], initial=1, aps=[p0:(OR (AND (GEQ s14 1) (GEQ s30 1) (GEQ s46 1) (GEQ s58 1)) (AND (GEQ s7 1) (GEQ s23 1) (GEQ s38 1) (GEQ s59 1)) (AND (GEQ s15 1) (GEQ s27 1) (GEQ s44 1)...], nbAcceptance=1, properties=[trans-labels, explicit-labels, trans-acc, complete, deterministic, no-univ-branch, unambiguous, semi-deterministic, stutter-sensitive, terminal, very-weak, weak, inherently-weak], stateDesc=[null, null, null][false, false, false]]
Entered a terminal (fully accepting) state of product in 12 steps with 0 reset in 0 ms.
FORMULA Sudoku-COL-BN04-LTLFireability-07 FALSE TECHNIQUES STUTTER_TEST
Treatment of property Sudoku-COL-BN04-LTLFireability-07 finished in 234 ms.
Running Spot : cd /home/mcc/execution;'/home/mcc/BenchKit/bin//..//ltl2tgba' '--check=stutter' '--hoaf=tv' '-f' '!((X((G((G(p1)&&p1))||p0))&&X(p1)))'
Support contains 64 out of 64 places. Attempting structural reductions.
Starting structural reductions in LTL mode, iteration 0 : 64/64 places, 64/64 transitions.
Applied a total of 0 rules in 0 ms. Remains 64 /64 variables (removed 0) and now considering 64/64 (removed 0) transitions.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:46] [INFO ] Computed 24 place invariants in 0 ms
[2022-05-20 23:05:46] [INFO ] Implicit Places using invariants in 28 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:46] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:46] [INFO ] Implicit Places using invariants and state equation in 44 ms returned []
Implicit Place search using SMT with State Equation took 74 ms to find 0 implicit places.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:46] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:46] [INFO ] Dead Transitions using invariants and state equation in 47 ms found 0 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/64 places, 64/64 transitions.
Stuttering acceptance computed with spot in 115 ms :[true, (NOT p1), (NOT p1), (NOT p1)]
Running random walk in product with property : Sudoku-COL-BN04-LTLFireability-08 automaton TGBA Formula[mat=[[{ cond=true, acceptance={0} source=0 dest: 0}], [{ cond=true, acceptance={} source=1 dest: 2}], [{ cond=(NOT p1), acceptance={} source=2 dest: 0}, { cond=(AND p1 (NOT p0)), acceptance={} source=2 dest: 3}], [{ cond=(NOT p1), acceptance={} source=3 dest: 0}, { cond=p1, acceptance={} source=3 dest: 3}]], initial=1, aps=[p1:(OR (AND (GEQ s14 1) (GEQ s30 1) (GEQ s46 1) (GEQ s58 1)) (AND (GEQ s7 1) (GEQ s23 1) (GEQ s38 1) (GEQ s59 1)) (AND (GEQ s15 1) (GEQ s27 1) (GEQ s44 1)...], nbAcceptance=1, properties=[trans-labels, explicit-labels, trans-acc, deterministic, no-univ-branch, unambiguous, semi-deterministic, stutter-sensitive, terminal, very-weak, weak, inherently-weak], stateDesc=[null, null, null, null][false, false, false, false]]
Product exploration explored 100000 steps with 50000 reset in 623 ms.
Product exploration explored 100000 steps with 50000 reset in 655 ms.
Computed a total of 64 stabilizing places and 64 stable transitions
Complete graph has no SCC; deadlocks are unavoidable. place count 64 transition count 64
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge (and all enablings converge to false).
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge : F ( (Ga|G!a) & (Gb|G!b)...)
Knowledge obtained : [(AND p1 p0), (X p1), (X (NOT (AND p1 (NOT p0)))), true, (X (X p1)), (F (G (NOT p1))), (F (G (NOT p0)))]
False Knowledge obtained : []
Property proved to be true thanks to knowledge :(X (NOT (AND p1 (NOT p0))))
Knowledge based reduction with 7 factoid took 119 ms. Reduced automaton from 4 states, 6 edges and 2 AP to 1 states, 0 edges and 0 AP.
FORMULA Sudoku-COL-BN04-LTLFireability-08 TRUE TECHNIQUES KNOWLEDGE
Treatment of property Sudoku-COL-BN04-LTLFireability-08 finished in 1701 ms.
Running Spot : cd /home/mcc/execution;'/home/mcc/BenchKit/bin//..//ltl2tgba' '--check=stutter' '--hoaf=tv' '-f' '!(G((X(G(p0))||G(p0))))'
Support contains 64 out of 64 places. Attempting structural reductions.
Starting structural reductions in LTL mode, iteration 0 : 64/64 places, 64/64 transitions.
Applied a total of 0 rules in 1 ms. Remains 64 /64 variables (removed 0) and now considering 64/64 (removed 0) transitions.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:48] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:48] [INFO ] Implicit Places using invariants in 30 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:48] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:48] [INFO ] Implicit Places using invariants and state equation in 41 ms returned []
Implicit Place search using SMT with State Equation took 72 ms to find 0 implicit places.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:48] [INFO ] Computed 24 place invariants in 0 ms
[2022-05-20 23:05:48] [INFO ] Dead Transitions using invariants and state equation in 46 ms found 0 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/64 places, 64/64 transitions.
Stuttering acceptance computed with spot in 74 ms :[true, (NOT p0), (NOT p0)]
Running random walk in product with property : Sudoku-COL-BN04-LTLFireability-09 automaton TGBA Formula[mat=[[{ cond=true, acceptance={0} source=0 dest: 0}], [{ cond=true, acceptance={} source=1 dest: 2}], [{ cond=(NOT p0), acceptance={} source=2 dest: 0}, { cond=p0, acceptance={} source=2 dest: 2}]], initial=1, aps=[p0:(OR (AND (GEQ s14 1) (GEQ s30 1) (GEQ s46 1) (GEQ s58 1)) (AND (GEQ s7 1) (GEQ s23 1) (GEQ s38 1) (GEQ s59 1)) (AND (GEQ s15 1) (GEQ s27 1) (GEQ s44 1)...], nbAcceptance=1, properties=[trans-labels, explicit-labels, trans-acc, complete, deterministic, no-univ-branch, unambiguous, semi-deterministic, stutter-sensitive, terminal, very-weak, weak, inherently-weak], stateDesc=[null, null, null][false, false, false]]
Entered a terminal (fully accepting) state of product in 12 steps with 0 reset in 0 ms.
FORMULA Sudoku-COL-BN04-LTLFireability-09 FALSE TECHNIQUES STUTTER_TEST
Treatment of property Sudoku-COL-BN04-LTLFireability-09 finished in 207 ms.
Running Spot : cd /home/mcc/execution;'/home/mcc/BenchKit/bin//..//ltl2tgba' '--check=stutter' '--hoaf=tv' '-f' '!(X(X((X(G(p0))||X(F(X(G(p0))))))))'
Support contains 64 out of 64 places. Attempting structural reductions.
Starting structural reductions in SI_LTL mode, iteration 0 : 64/64 places, 64/64 transitions.
Applied a total of 0 rules in 3 ms. Remains 64 /64 variables (removed 0) and now considering 64/64 (removed 0) transitions.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:48] [INFO ] Computed 24 place invariants in 2 ms
[2022-05-20 23:05:48] [INFO ] Implicit Places using invariants in 30 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:48] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:48] [INFO ] Implicit Places using invariants and state equation in 48 ms returned []
Implicit Place search using SMT with State Equation took 79 ms to find 0 implicit places.
[2022-05-20 23:05:48] [INFO ] Redundant transitions in 1 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:48] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:48] [INFO ] Dead Transitions using invariants and state equation in 45 ms found 0 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/64 places, 64/64 transitions.
Stuttering acceptance computed with spot in 36 ms :[(NOT p0)]
Running random walk in product with property : Sudoku-COL-BN04-LTLFireability-10 automaton TGBA Formula[mat=[[{ cond=p0, acceptance={} source=0 dest: 0}, { cond=(NOT p0), acceptance={0} source=0 dest: 0}]], initial=0, aps=[p0:(AND (OR (LT s14 1) (LT s30 1) (LT s46 1) (LT s58 1)) (OR (LT s7 1) (LT s23 1) (LT s38 1) (LT s59 1)) (OR (LT s15 1) (LT s27 1) (LT s44 1) (LT s51 1)) ...], nbAcceptance=1, properties=[trans-labels, explicit-labels, trans-acc, complete, deterministic, no-univ-branch, unambiguous, semi-deterministic, stutter-invariant], stateDesc=[null][true]]
Product exploration explored 100000 steps with 7171 reset in 633 ms.
Product exploration explored 100000 steps with 7173 reset in 643 ms.
Computed a total of 64 stabilizing places and 64 stable transitions
Complete graph has no SCC; deadlocks are unavoidable. place count 64 transition count 64
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge (and all enablings converge to false).
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge : F ( (Ga|G!a) & (Gb|G!b)...)
Knowledge obtained : [(NOT p0), (X (NOT p0)), (X (X (NOT p0))), (F (G p0))]
False Knowledge obtained : []
Property proved to be true thanks to knowledge :(F (G p0))
Knowledge based reduction with 4 factoid took 93 ms. Reduced automaton from 1 states, 2 edges and 1 AP to 1 states, 0 edges and 0 AP.
FORMULA Sudoku-COL-BN04-LTLFireability-10 TRUE TECHNIQUES KNOWLEDGE
Treatment of property Sudoku-COL-BN04-LTLFireability-10 finished in 1608 ms.
Running Spot : cd /home/mcc/execution;'/home/mcc/BenchKit/bin//..//ltl2tgba' '--check=stutter' '--hoaf=tv' '-f' '!(X((F(F(G(X(X(p1)))))||p0)))'
Support contains 64 out of 64 places. Attempting structural reductions.
Starting structural reductions in LTL mode, iteration 0 : 64/64 places, 64/64 transitions.
Applied a total of 0 rules in 1 ms. Remains 64 /64 variables (removed 0) and now considering 64/64 (removed 0) transitions.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:50] [INFO ] Computed 24 place invariants in 0 ms
[2022-05-20 23:05:50] [INFO ] Implicit Places using invariants in 27 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:50] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:50] [INFO ] Implicit Places using invariants and state equation in 42 ms returned []
Implicit Place search using SMT with State Equation took 70 ms to find 0 implicit places.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:50] [INFO ] Computed 24 place invariants in 2 ms
[2022-05-20 23:05:50] [INFO ] Dead Transitions using invariants and state equation in 46 ms found 0 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/64 places, 64/64 transitions.
Stuttering acceptance computed with spot in 103 ms :[(AND (NOT p0) (NOT p1)), (NOT p1), (AND (NOT p0) (NOT p1))]
Running random walk in product with property : Sudoku-COL-BN04-LTLFireability-12 automaton TGBA Formula[mat=[[{ cond=(NOT p0), acceptance={} source=0 dest: 1}], [{ cond=p1, acceptance={} source=1 dest: 1}, { cond=(NOT p1), acceptance={0} source=1 dest: 1}], [{ cond=true, acceptance={} source=2 dest: 0}]], initial=2, aps=[p0:(OR (AND (GEQ s14 1) (GEQ s30 1) (GEQ s46 1) (GEQ s58 1)) (AND (GEQ s7 1) (GEQ s23 1) (GEQ s38 1) (GEQ s59 1)) (AND (GEQ s15 1) (GEQ s27 1) (GEQ s44 1)...], nbAcceptance=1, properties=[trans-labels, explicit-labels, trans-acc, deterministic, no-univ-branch, unambiguous, semi-deterministic, stutter-sensitive], stateDesc=[null, null, null][false, false, false]]
Product exploration explored 100000 steps with 50000 reset in 597 ms.
Product exploration explored 100000 steps with 50000 reset in 616 ms.
Computed a total of 64 stabilizing places and 64 stable transitions
Complete graph has no SCC; deadlocks are unavoidable. place count 64 transition count 64
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge (and all enablings converge to false).
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge : F ( (Ga|G!a) & (Gb|G!b)...)
Knowledge obtained : [(AND p0 (NOT p1)), (X p0), (X (X (NOT p1))), (F (G (NOT p0))), (F (G p1))]
False Knowledge obtained : []
Property proved to be true thanks to knowledge :(X p0)
Knowledge based reduction with 5 factoid took 93 ms. Reduced automaton from 3 states, 4 edges and 2 AP to 1 states, 0 edges and 0 AP.
FORMULA Sudoku-COL-BN04-LTLFireability-12 TRUE TECHNIQUES KNOWLEDGE
Treatment of property Sudoku-COL-BN04-LTLFireability-12 finished in 1612 ms.
Running Spot : cd /home/mcc/execution;'/home/mcc/BenchKit/bin//..//ltl2tgba' '--check=stutter' '--hoaf=tv' '-f' '!(X(X(F(X(p0)))))'
Support contains 64 out of 64 places. Attempting structural reductions.
Starting structural reductions in LTL mode, iteration 0 : 64/64 places, 64/64 transitions.
Applied a total of 0 rules in 1 ms. Remains 64 /64 variables (removed 0) and now considering 64/64 (removed 0) transitions.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:51] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:51] [INFO ] Implicit Places using invariants in 26 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:51] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:51] [INFO ] Implicit Places using invariants and state equation in 38 ms returned []
Implicit Place search using SMT with State Equation took 66 ms to find 0 implicit places.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:51] [INFO ] Computed 24 place invariants in 2 ms
[2022-05-20 23:05:52] [INFO ] Dead Transitions using invariants and state equation in 48 ms found 0 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/64 places, 64/64 transitions.
Stuttering acceptance computed with spot in 150 ms :[(NOT p0), (NOT p0), (NOT p0), (NOT p0)]
Running random walk in product with property : Sudoku-COL-BN04-LTLFireability-14 automaton TGBA Formula[mat=[[{ cond=(NOT p0), acceptance={0} source=0 dest: 0}], [{ cond=true, acceptance={} source=1 dest: 0}], [{ cond=true, acceptance={} source=2 dest: 3}], [{ cond=true, acceptance={} source=3 dest: 1}]], initial=2, aps=[p0:(OR (AND (GEQ s14 1) (GEQ s30 1) (GEQ s46 1) (GEQ s58 1)) (AND (GEQ s7 1) (GEQ s23 1) (GEQ s38 1) (GEQ s59 1)) (AND (GEQ s15 1) (GEQ s27 1) (GEQ s44 1)...], nbAcceptance=1, properties=[trans-labels, explicit-labels, trans-acc, deterministic, no-univ-branch, unambiguous, semi-deterministic, stutter-sensitive, very-weak, weak, inherently-weak], stateDesc=[null, null, null, null][false, false, false, false]]
Product exploration explored 100000 steps with 25000 reset in 755 ms.
Product exploration explored 100000 steps with 25000 reset in 769 ms.
Computed a total of 64 stabilizing places and 64 stable transitions
Complete graph has no SCC; deadlocks are unavoidable. place count 64 transition count 64
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge (and all enablings converge to false).
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge : F ( (Ga|G!a) & (Gb|G!b)...)
Knowledge obtained : [p0, true, (F (G (NOT p0)))]
False Knowledge obtained : []
Knowledge based reduction with 3 factoid took 836 ms. Reduced automaton from 4 states, 4 edges and 1 AP to 4 states, 4 edges and 1 AP.
Stuttering acceptance computed with spot in 134 ms :[(NOT p0), (NOT p0), (NOT p0), (NOT p0)]
Finished random walk after 12 steps, including 0 resets, run visited all 1 properties in 2 ms. (steps per millisecond=6 )
Knowledge obtained : [p0, true, (F (G (NOT p0)))]
False Knowledge obtained : [(F (NOT p0))]
Knowledge based reduction with 3 factoid took 110 ms. Reduced automaton from 4 states, 4 edges and 1 AP to 4 states, 4 edges and 1 AP.
Stuttering acceptance computed with spot in 129 ms :[(NOT p0), (NOT p0), (NOT p0), (NOT p0)]
Stuttering acceptance computed with spot in 122 ms :[(NOT p0), (NOT p0), (NOT p0), (NOT p0)]
Support contains 64 out of 64 places. Attempting structural reductions.
Starting structural reductions in LTL mode, iteration 0 : 64/64 places, 64/64 transitions.
Applied a total of 0 rules in 1 ms. Remains 64 /64 variables (removed 0) and now considering 64/64 (removed 0) transitions.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:55] [INFO ] Computed 24 place invariants in 2 ms
[2022-05-20 23:05:55] [INFO ] Implicit Places using invariants in 30 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:55] [INFO ] Computed 24 place invariants in 2 ms
[2022-05-20 23:05:55] [INFO ] Implicit Places using invariants and state equation in 44 ms returned []
Implicit Place search using SMT with State Equation took 76 ms to find 0 implicit places.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:55] [INFO ] Computed 24 place invariants in 2 ms
[2022-05-20 23:05:55] [INFO ] Dead Transitions using invariants and state equation in 60 ms found 0 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/64 places, 64/64 transitions.
Computed a total of 64 stabilizing places and 64 stable transitions
Complete graph has no SCC; deadlocks are unavoidable. place count 64 transition count 64
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge (and all enablings converge to false).
Detected that all paths lead to deadlock. Applying this knowledge to assert that all AP eventually converge : F ( (Ga|G!a) & (Gb|G!b)...)
Knowledge obtained : [p0, true, (F (G (NOT p0)))]
False Knowledge obtained : []
Knowledge based reduction with 3 factoid took 115 ms. Reduced automaton from 4 states, 4 edges and 1 AP to 4 states, 4 edges and 1 AP.
Stuttering acceptance computed with spot in 143 ms :[(NOT p0), (NOT p0), (NOT p0), (NOT p0)]
Finished random walk after 14 steps, including 0 resets, run visited all 1 properties in 2 ms. (steps per millisecond=7 )
Knowledge obtained : [p0, true, (F (G (NOT p0)))]
False Knowledge obtained : [(F (NOT p0))]
Knowledge based reduction with 3 factoid took 113 ms. Reduced automaton from 4 states, 4 edges and 1 AP to 4 states, 4 edges and 1 AP.
Stuttering acceptance computed with spot in 313 ms :[(NOT p0), (NOT p0), (NOT p0), (NOT p0)]
Stuttering acceptance computed with spot in 131 ms :[(NOT p0), (NOT p0), (NOT p0), (NOT p0)]
Stuttering acceptance computed with spot in 356 ms :[(NOT p0), (NOT p0), (NOT p0), (NOT p0)]
Product exploration explored 100000 steps with 25000 reset in 772 ms.
Product exploration explored 100000 steps with 25000 reset in 806 ms.
Applying partial POR strategy [true, false, false, false]
Stuttering acceptance computed with spot in 131 ms :[(NOT p0), (NOT p0), (NOT p0), (NOT p0)]
Support contains 64 out of 64 places. Attempting structural reductions.
Starting structural reductions in SI_LTL mode, iteration 0 : 64/64 places, 64/64 transitions.
Applied a total of 0 rules in 1 ms. Remains 64 /64 variables (removed 0) and now considering 64/64 (removed 0) transitions.
[2022-05-20 23:05:58] [INFO ] Redundant transitions in 1 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:05:58] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:05:58] [INFO ] Dead Transitions using invariants and state equation in 49 ms found 0 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/64 places, 64/64 transitions.
Product exploration explored 100000 steps with 25000 reset in 998 ms.
Product exploration explored 100000 steps with 25000 reset in 974 ms.
Built C files in :
/tmp/ltsmin11187710969600031641
[2022-05-20 23:06:00] [INFO ] Built C files in 15ms conformant to PINS (ltsmin variant)in folder :/tmp/ltsmin11187710969600031641
Running compilation step : cd /tmp/ltsmin11187710969600031641;'/home/mcc/BenchKit/itstools/plugins/fr.lip6.move.gal.ltsmin.binaries_1.0.0.202205111006/bin/limit_time.pl' '3' 'gcc' '-c' '-I/home/mcc/BenchKit/itstools/plugins/fr.lip6.move.gal.ltsmin.binaries_1.0.0.202205111006/bin/include/' '-I.' '-std=c99' '-fPIC' '-O0' 'model.c'
Compilation finished in 306 ms.
Running link step : cd /tmp/ltsmin11187710969600031641;'gcc' '-shared' '-o' 'gal.so' 'model.o'
Link finished in 36 ms.
Running LTSmin : cd /tmp/ltsmin11187710969600031641;'/home/mcc/BenchKit/itstools/plugins/fr.lip6.move.gal.ltsmin.binaries_1.0.0.202205111006/bin/pins2lts-mc-linux64' './gal.so' '--threads=8' '--when' '--hoa' '/tmp/stateBased12358247784023371042.hoa' '--buchi-type=spotba'
LTSmin run took 2409 ms.
FORMULA Sudoku-COL-BN04-LTLFireability-14 TRUE TECHNIQUES EXPLICIT LTSMIN SAT_SMT
Treatment of property Sudoku-COL-BN04-LTLFireability-14 finished in 11620 ms.
Running Spot : cd /home/mcc/execution;'/home/mcc/BenchKit/bin//..//ltl2tgba' '--check=stutter' '--hoaf=tv' '-f' '!(G(X(X(p0))))'
Support contains 64 out of 64 places. Attempting structural reductions.
Starting structural reductions in LTL mode, iteration 0 : 64/64 places, 64/64 transitions.
Applied a total of 0 rules in 1 ms. Remains 64 /64 variables (removed 0) and now considering 64/64 (removed 0) transitions.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:06:04] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:06:04] [INFO ] Implicit Places using invariants in 35 ms returned []
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:06:04] [INFO ] Computed 24 place invariants in 2 ms
[2022-05-20 23:06:04] [INFO ] Implicit Places using invariants and state equation in 56 ms returned []
Implicit Place search using SMT with State Equation took 92 ms to find 0 implicit places.
// Phase 1: matrix 64 rows 64 cols
[2022-05-20 23:06:04] [INFO ] Computed 24 place invariants in 1 ms
[2022-05-20 23:06:04] [INFO ] Dead Transitions using invariants and state equation in 44 ms found 0 transitions.
Finished structural reductions, in 1 iterations. Remains : 64/64 places, 64/64 transitions.
Stuttering acceptance computed with spot in 141 ms :[true, (NOT p0), (NOT p0), (NOT p0)]
Running random walk in product with property : Sudoku-COL-BN04-LTLFireability-15 automaton TGBA Formula[mat=[[{ cond=true, acceptance={0} source=0 dest: 0}], [{ cond=(NOT p0), acceptance={} source=1 dest: 0}, { cond=p0, acceptance={} source=1 dest: 1}], [{ cond=true, acceptance={} source=2 dest: 1}], [{ cond=true, acceptance={} source=3 dest: 2}]], initial=3, aps=[p0:(OR (AND (GEQ s14 1) (GEQ s30 1) (GEQ s46 1) (GEQ s58 1)) (AND (GEQ s7 1) (GEQ s23 1) (GEQ s38 1) (GEQ s59 1)) (AND (GEQ s15 1) (GEQ s27 1) (GEQ s44 1)...], nbAcceptance=1, properties=[trans-labels, explicit-labels, trans-acc, complete, deterministic, no-univ-branch, unambiguous, semi-deterministic, stutter-sensitive, terminal, very-weak, weak, inherently-weak], stateDesc=[null, null, null, null][false, false, false, false]]
Entered a terminal (fully accepting) state of product in 14 steps with 0 reset in 1 ms.
FORMULA Sudoku-COL-BN04-LTLFireability-15 FALSE TECHNIQUES STUTTER_TEST
Treatment of property Sudoku-COL-BN04-LTLFireability-15 finished in 1129 ms.
All properties solved by simple procedures.
Total runtime 27196 ms.
BK_STOP 1653087965117
--------------------
content from stderr:
+ export LANG=C
+ LANG=C
+ export BINDIR=/home/mcc/BenchKit/bin//../
+ BINDIR=/home/mcc/BenchKit/bin//../
++ pwd
+ export MODEL=/home/mcc/execution
+ MODEL=/home/mcc/execution
+ [[ LTLFireability = StateSpace ]]
+ /home/mcc/BenchKit/bin//..//runeclipse.sh /home/mcc/execution LTLFireability -its -ltsmin -greatspnpath /home/mcc/BenchKit/bin//..//greatspn/ -order META -manyOrder -smt -timeout 3600
+ ulimit -s 65536
+ export PYTHONPATH=/usr/lib/python3.9/site-packages/
+ PYTHONPATH=/usr/lib/python3.9/site-packages/
+ export LD_LIBRARY_PATH=/usr/local/lib:
+ LD_LIBRARY_PATH=/usr/local/lib:
+ [[ -z '' ]]
+ export LTSMIN_MEM_SIZE=8589934592
+ LTSMIN_MEM_SIZE=8589934592
++ sed s/.jar//
++ perl -pe 's/.*\.//g'
++ ls /home/mcc/BenchKit/bin//..//itstools/plugins/fr.lip6.move.gal.application.pnmcc_1.0.0.202205111006.jar
+ VERSION=202205111006
+ echo 'Running Version 202205111006'
+ /home/mcc/BenchKit/bin//..//itstools/its-tools -data @none -pnfolder /home/mcc/execution -examination LTLFireability -spotpath /home/mcc/BenchKit/bin//..//ltlfilt -z3path /home/mcc/BenchKit/bin//..//z3/bin/z3 -yices2path /home/mcc/BenchKit/bin//..//yices/bin/yices -its -ltsmin -greatspnpath /home/mcc/BenchKit/bin//..//greatspn/ -order META -manyOrder -smt -timeout 3600 -vmargs -Dosgi.locking=none -Declipse.stateSaveDelayInterval=-1 -Dosgi.configuration.area=@none -Xss128m -Xms40m -Xmx8192m
Sequence of Actions to be Executed by the VM
This is useful if one wants to reexecute the tool in the VM from the submitted image disk.
set -x
# this is for BenchKit: configuration of major elements for the test
export BK_INPUT="Sudoku-COL-BN04"
export BK_EXAMINATION="LTLFireability"
export BK_TOOL="itstools"
export BK_RESULT_DIR="/tmp/BK_RESULTS/OUTPUTS"
export BK_TIME_CONFINEMENT="3600"
export BK_MEMORY_CONFINEMENT="16384"
export BK_BIN_PATH="/home/mcc/BenchKit/bin/"
# this is specific to your benchmark or test
export BIN_DIR="$HOME/BenchKit/bin"
# remove the execution directoty if it exists (to avoid increse of .vmdk images)
if [ -d execution ] ; then
rm -rf execution
fi
# this is for BenchKit: explicit launching of the test
echo "====================================================================="
echo " Generated by BenchKit 2-4028"
echo " Executing tool itstools"
echo " Input is Sudoku-COL-BN04, examination is LTLFireability"
echo " Time confinement is $BK_TIME_CONFINEMENT seconds"
echo " Memory confinement is 16384 MBytes"
echo " Number of cores is 4"
echo " Run identifier is r258-tall-165303545400180"
echo "====================================================================="
echo
echo "--------------------"
echo "preparation of the directory to be used:"
tar xzf /home/mcc/BenchKit/INPUTS/Sudoku-COL-BN04.tgz
mv Sudoku-COL-BN04 execution
cd execution
if [ "LTLFireability" = "ReachabilityDeadlock" ] || [ "LTLFireability" = "UpperBounds" ] || [ "LTLFireability" = "QuasiLiveness" ] || [ "LTLFireability" = "StableMarking" ] || [ "LTLFireability" = "Liveness" ] || [ "LTLFireability" = "OneSafe" ] || [ "LTLFireability" = "StateSpace" ]; then
rm -f GenericPropertiesVerdict.xml
fi
pwd
ls -lh
echo
echo "--------------------"
echo "content from stdout:"
echo
echo "=== Data for post analysis generated by BenchKit (invocation template)"
echo
if [ "LTLFireability" = "UpperBounds" ] ; then
echo "The expected result is a vector of positive values"
echo NUM_VECTOR
elif [ "LTLFireability" != "StateSpace" ] ; then
echo "The expected result is a vector of booleans"
echo BOOL_VECTOR
else
echo "no data necessary for post analysis"
fi
echo
if [ -f "LTLFireability.txt" ] ; then
echo "here is the order used to build the result vector(from text file)"
for x in $(grep Property LTLFireability.txt | cut -d ' ' -f 2 | sort -u) ; do
echo "FORMULA_NAME $x"
done
elif [ -f "LTLFireability.xml" ] ; then # for cunf (txt files deleted;-)
echo echo "here is the order used to build the result vector(from xml file)"
for x in $(grep '
echo "FORMULA_NAME $x"
done
elif [ "LTLFireability" = "ReachabilityDeadlock" ] || [ "LTLFireability" = "QuasiLiveness" ] || [ "LTLFireability" = "StableMarking" ] || [ "LTLFireability" = "Liveness" ] || [ "LTLFireability" = "OneSafe" ] ; then
echo "FORMULA_NAME LTLFireability"
fi
echo
echo "=== Now, execution of the tool begins"
echo
echo -n "BK_START "
date -u +%s%3N
echo
timeout -s 9 $BK_TIME_CONFINEMENT bash -c "/home/mcc/BenchKit/BenchKit_head.sh 2> STDERR ; echo ; echo -n \"BK_STOP \" ; date -u +%s%3N"
if [ $? -eq 137 ] ; then
echo
echo "BK_TIME_CONFINEMENT_REACHED"
fi
echo
echo "--------------------"
echo "content from stderr:"
echo
cat STDERR ;

